
If you are studying algebra 1, you know how important it is to have access to the right resources for learning and practicing. One resource that can greatly help you in your studies is the Glencoe Algebra 1 textbook. Glencoe Algebra 1 provides a comprehensive coverage of all the topics and concepts in algebra 1, making it an invaluable tool for students.
Chapter 5 of Glencoe Algebra 1 focuses on the topic of linear equations and inequalities. This chapter explores different methods for solving linear equations and inequalities, including graphing, substitution, and elimination. It also covers topics such as systems of linear equations, absolute value equations and inequalities, and compound inequalities. Mastering the concepts and techniques in this chapter is essential for success in algebra 1 and beyond.
To aid in your learning, Glencoe Algebra 1 provides answers to all the exercises and problems in the textbook. These answers can be found in the back of the book and can serve as a valuable tool for checking your work and understanding the material. Having access to the chapter 5 answers can help you gauge your progress and identify areas where you may need additional practice or clarification.
In this article, we will provide an overview of the chapter 5 Glencoe Algebra 1 answers. We will highlight some of the key concepts and techniques covered in the chapter and discuss how the answers can be used to support your learning and enhance your understanding of algebra 1. Whether you are a student looking to check your work or a teacher seeking additional resources for your students, the chapter 5 Glencoe Algebra 1 answers can be a valuable tool in your mathematical journey.
Understanding Chapter 5 Glencoe Algebra 1 Answers
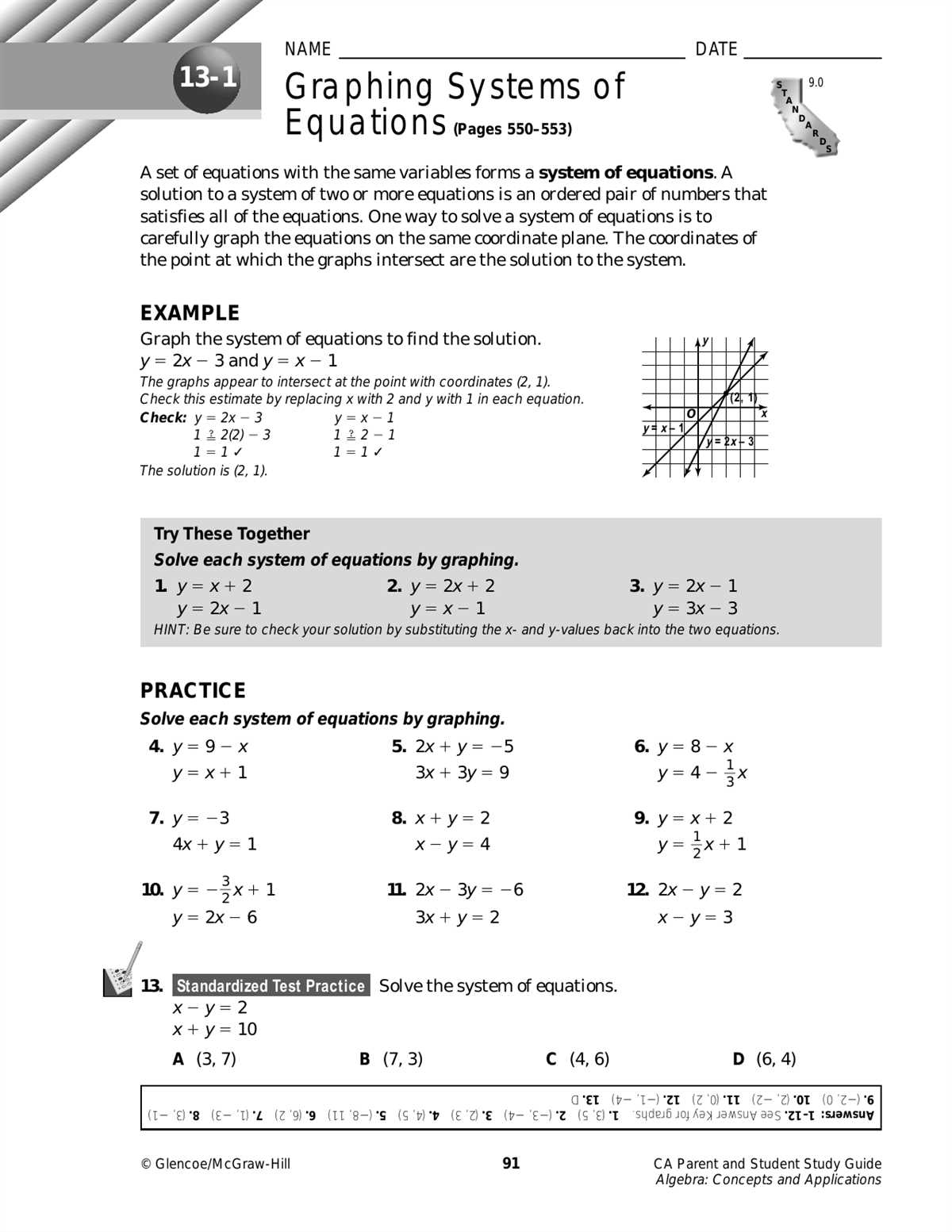
Chapter 5 in Glencoe Algebra 1 is an important section that focuses on the topic of quadratic functions and equations. In this chapter, students are introduced to the concept of a quadratic function, which is a function that can be written in the form f(x) = ax^2 + bx + c. The chapter explores various aspects of quadratic functions, including their graphs, vertex form, and different methods for solving quadratic equations.
One of the key objectives of Chapter 5 is to help students understand how to find the solutions to quadratic equations. This involves learning different methods, such as factoring, completing the square, and using the quadratic formula. The chapter provides step-by-step instructions and examples to guide students through each method, helping them develop a solid foundation in solving quadratic equations.
Throughout Chapter 5, Glencoe Algebra 1 provides practice problems and exercises to reinforce the concepts and skills learned. Students are encouraged to apply their understanding of quadratic functions and equations to real-world scenarios and problem-solving situations. The chapter also includes opportunities for students to explore and analyze quadratic functions graphically, allowing them to make connections between algebraic representations and visual representations.
By the end of Chapter 5, students should have a strong grasp of quadratic functions and equations. They should be able to identify key features of quadratic graphs, solve quadratic equations using various methods, and apply their knowledge to solve real-world problems. The chapter concludes with a review section that allows students to test their understanding and prepare for future assessments. Overall, Chapter 5 in Glencoe Algebra 1 provides a comprehensive introduction to quadratic functions and equations, laying the groundwork for more advanced topics in algebra.
The Importance of Chapter 5 in Glencoe Algebra 1
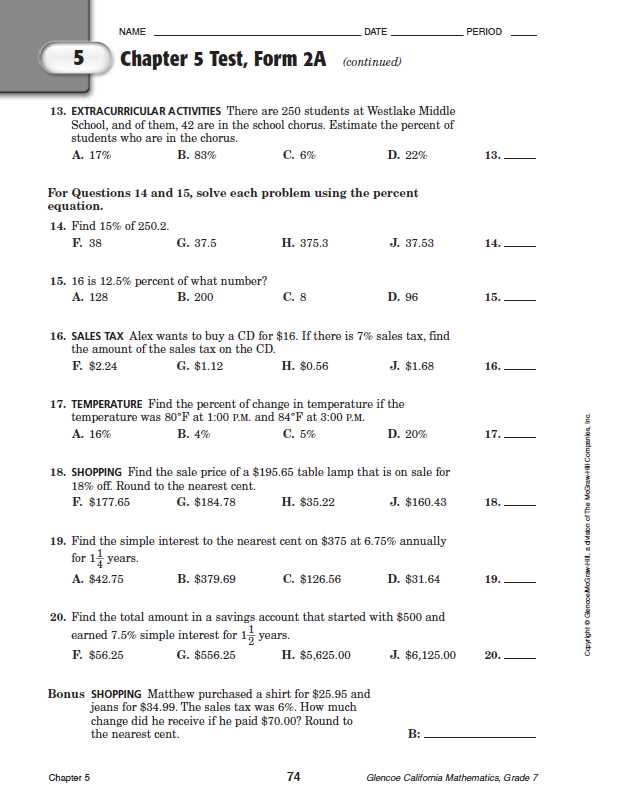
Chapter 5 of Glencoe Algebra 1 is a crucial part of the curriculum as it delves into the topic of linear equations and inequalities. In this chapter, students are introduced to the fundamental concepts and techniques required to solve equations and understand the behavior of linear functions.
One of the key concepts covered in Chapter 5 is the slope-intercept form of a linear equation, which is represented as y = mx + b. This form allows students to easily identify the slope (m) and y-intercept (b) of a line, enabling them to graph and analyze linear functions efficiently. Mastering this form is essential for solving real-world problems and understanding the relationship between variables.
Another important topic in Chapter 5 is solving systems of linear equations. Students learn different methods, such as substitution and elimination, to find the values of x and y that satisfy two or more equations simultaneously. This skill is particularly valuable in various fields, including engineering, economics, and physics, where multiple variables are involved.
Furthermore, Chapter 5 introduces the concept of inequalities and teaches students how to solve and graph linear inequalities. This knowledge is vital for understanding and analyzing real-world situations that involve constraints and limitations, such as budgeting, resource allocation, and optimization problems.
In summary, Chapter 5 in Glencoe Algebra 1 plays a crucial role in building a strong foundation in algebra. It equips students with the necessary problem-solving skills and mathematical tools to tackle linear equations, systems of equations, and inequalities, which are essential for success in higher-level math courses and practical applications.
Exploring the Concepts in Chapter 5 Glencoe Algebra 1
The concepts covered in Chapter 5 of Glencoe Algebra 1 delve into the world of quadratic equations and functions. This chapter introduces students to the fundamental principles of quadratic equations and provides them with the tools and techniques necessary to solve various quadratic equations. Students will also explore the connection between quadratic equations and the graphical representations of parabolas.
One of the key concepts in this chapter is the quadratic formula. This powerful formula allows students to find the solutions to quadratic equations of the form ax^2 + bx + c = 0, where a, b, and c are constants. The quadratic formula states that the solutions are given by x = (-b ± √(b^2 – 4ac))/(2a). By utilizing this formula, students can solve quadratic equations with ease.
The chapter also introduces students to the concept of factoring quadratic expressions. Factoring involves breaking down a quadratic expression into its simpler components, which can then be multiplied together to obtain the original expression. Factoring quadratic expressions is crucial when solving quadratic equations, as it allows students to rewrite the equation in a factored form, making it easier to identify the solutions.
Furthermore, Chapter 5 explores the relationship between quadratic equations and the graph of a parabola. Students will learn how to graph quadratic functions using key points, such as the vertex, axis of symmetry, and the y-intercept. They will also gain an understanding of how changes in the coefficients of a quadratic equation affect the shape and position of the corresponding parabola.
In conclusion, Chapter 5 of Glencoe Algebra 1 introduces students to the intricacies of quadratic equations and functions. It equips students with the necessary tools, such as the quadratic formula and factoring techniques, to solve various types of quadratic equations. Additionally, students will gain a deeper understanding of the connection between quadratic equations and the graphical representations of parabolas. Mastery of the concepts covered in this chapter will provide students with a solid foundation in algebraic reasoning and problem-solving skills.
Step-by-Step Solutions to Chapter 5 Problems
In Chapter 5 of Glencoe Algebra 1, students explore the concept of linear equations and inequalities. They learn how to solve equations and inequalities using various methods such as graphing, substitution, and elimination. This chapter also introduces the concept of systems of equations and how to solve them using different techniques.
To help students understand and master the material, Glencoe Algebra 1 provides detailed step-by-step solutions to the problems in Chapter 5. These solutions guide students through the solving process, explaining each step along the way. Students can refer to these solutions to check their answers, learn from their mistakes, and improve their understanding of the concepts.
The step-by-step solutions start by identifying the problem and its given information. Then, they explain the method or strategy to be used in solving the equation or inequality. The solutions provide a clear and concise description of each step, making it easier for students to follow and comprehend. Intermediate steps are highlighted, and explanations are given to help students understand the reasoning behind each step.
In addition to providing solutions to the individual problems, Glencoe Algebra 1 also offers strategies and tips for solving different types of equations and inequalities. These strategies help students develop problem-solving skills and learn to approach different types of problems effectively.
Overall, the step-by-step solutions in Chapter 5 of Glencoe Algebra 1 are a valuable resource for students. They provide clear explanations, guide students through the solving process, and help them develop a deeper understanding of linear equations and inequalities. By using these solutions, students can improve their problem-solving skills and succeed in their algebra studies.
Common Mistakes to Avoid in Chapter 5 Glencoe Algebra 1

Chapter 5 of Glencoe Algebra 1 covers various topics such as solving quadratic equations, factoring, and solving systems of equations. While studying this chapter, it is important to be aware of common mistakes that students often make. By avoiding these mistakes, you can improve your understanding of the concepts and achieve better results in your algebra exams.
1. Forgetting to Apply the Distributive Property
One common mistake that students make is forgetting to apply the distributive property when simplifying expressions or solving equations. It is crucial to remember that when there is a multiplication or division outside parentheses, it needs to be distributed to every term inside the parentheses.
2. Not Checking for Extraneous Solutions
Another mistake to avoid is not checking for extraneous solutions. When solving equations, it is important to check the solutions obtained by substituting them back into the original equation. Sometimes, a solution may satisfy the equation but not the domain of the problem. These solutions need to be eliminated as they are not valid.
3. Incorrectly Applying the Zero Product Property
The Zero Product Property states that if the product of two or more factors is zero, then at least one of the factors must be zero. However, students often mistakenly assume that if an equation equals zero, each factor must also be zero. It is important to apply the Zero Product Property correctly to avoid incorrect solutions.
4. Confusing the Steps for Factoring Quadratic Equations

Factoring quadratic equations can be challenging, and students often confuse the steps involved in the process. It is crucial to practice factoring techniques and understand the difference between a perfect square trinomial, a difference of squares, and a general trinomial. Paying attention to the terms and signs in the equations can help avoid these common mistakes.
5. Misinterpreting Word Problems
Word problems can be tricky, and students may misinterpret the information given in the problem. To avoid mistakes, it is important to carefully read and understand the problem before attempting to solve it. Identifying the given variables, setting up equations correctly, and solving step-by-step can help prevent errors in word problem solving.
Avoiding these common mistakes will help you master the concepts covered in Chapter 5 of Glencoe Algebra 1. Practice regularly, seek clarification when needed, and be mindful of these errors to improve your performance in algebraic problem-solving.
Using Chapter 5 Glencoe Algebra 1 Answers to Improve Performance
When studying algebra, many students find themselves struggling to understand and apply the concepts taught in class. One resource that can be particularly helpful in this situation is the answers provided in the Glencoe Algebra 1 textbook. By using the Chapter 5 Glencoe Algebra 1 answers, students can improve their performance and gain a deeper understanding of the material.
Check answers and identify mistakes: One of the primary benefits of using the Chapter 5 Glencoe Algebra 1 answers is the ability to check answers and identify any mistakes made during practice or homework assignments. By comparing their answers to the correct ones, students can pinpoint areas where they may be making errors and work to correct them.
Gain a better understanding: In addition to checking answers, using the Chapter 5 Glencoe Algebra 1 answers allows students to gain a better understanding of the concepts being taught. By comparing their steps and solutions to the provided answers, students can see where they may have taken a different approach or made an error in their calculations. This process of self-correction can help clarify misconceptions and solidify understanding.
Practice effectively: By using the Chapter 5 Glencoe Algebra 1 answers, students can practice effectively and efficiently. As they work through practice problems, they can refer to the answers to check their progress and ensure they are on the right track. This can help students avoid wasting time on incorrect methods or solutions and focus their efforts where they are most needed.
Prepare for exams: The Chapter 5 Glencoe Algebra 1 answers can also be a valuable tool when preparing for exams. By practicing with the provided answers, students can familiarize themselves with the types of problems that may appear on the exam. This can help build confidence and reduce test anxiety, leading to improved overall performance.
In conclusion, using the Chapter 5 Glencoe Algebra 1 answers can greatly benefit students by allowing them to check their work, gain a better understanding of the material, practice effectively, and prepare for exams. By incorporating these answers into their study routine, students can improve their performance and achieve success in their algebra studies.