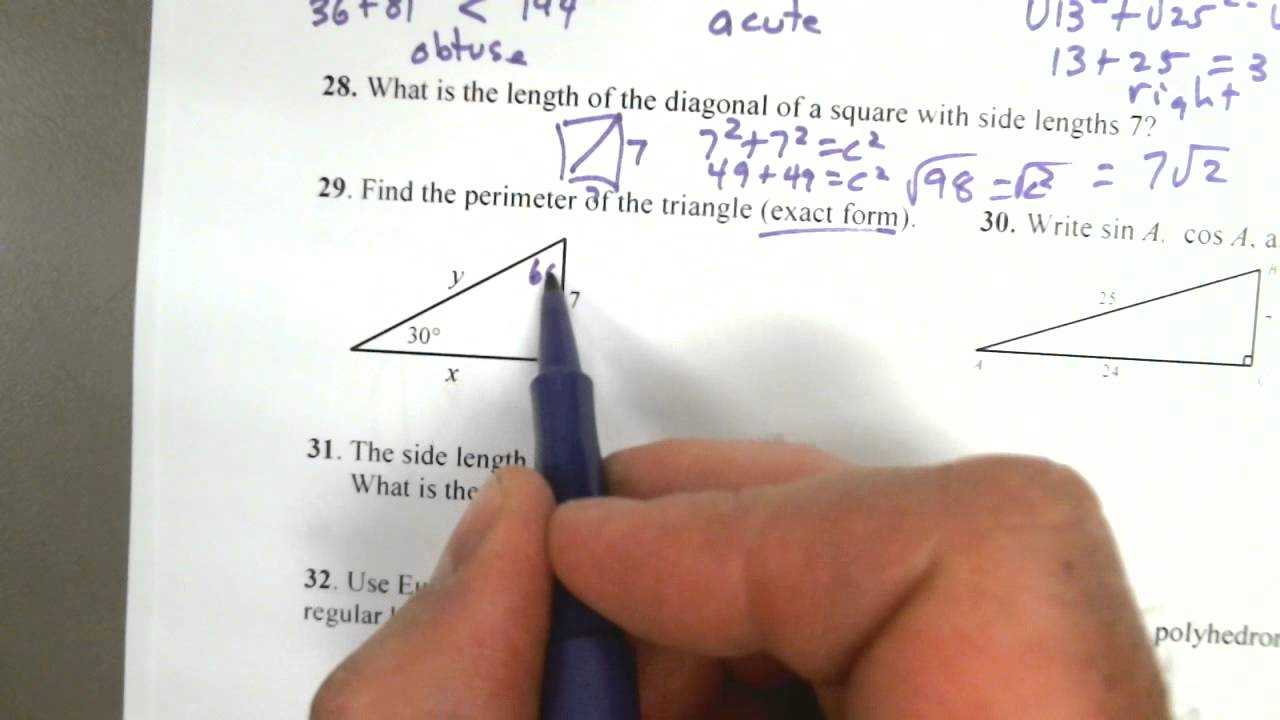
In this section, we will explore important concepts that lay the foundation for solving complex problems in a variety of mathematical fields. The focus will be on understanding core principles, applying various methods, and mastering essential techniques that are crucial for tackling advanced exercises. By diving into specific challenges, you will gain the skills necessary for success in both exams and real-world applications.
Critical Theories and Formulas
To succeed in this area, it is essential to grasp a range of fundamental theorems and equations. These form the building blocks for more intricate problem-solving tasks and are frequently tested. A solid understanding of these rules will make it easier to approach a wide variety of scenarios.
Essential Theorems
- Pythagorean Theorem – A fundamental relationship in right-angled triangles.
- Congruence Theorems – Key to proving geometric properties.
- Area and Volume Formulas – Critical for spatial reasoning and measurement.
Effective Problem-Solving Strategies
Successful problem-solving requires more than just memorization; it involves understanding the most efficient techniques to apply under different circumstances. One of the most useful approaches is breaking down complex problems into smaller, manageable parts.
Approaching Complex Problems

- Analyze the Problem: Understand the question before attempting to solve it.
- Identify Patterns: Look for connections that simplify the solution process.
- Check Results: Verify calculations and logic to ensure accuracy.
Test Preparation Tips
Familiarizing yourself with common problem types and practicing regularly can significantly improve your confidence and speed. Working on practice exercises allows you to refine your skills and become comfortable with different question formats.
Real-World Applications
The principles covered in this section extend far beyond the classroom. From architecture to engineering, the ability to solve these types of problems is critical for many professions. Understanding how theoretical concepts are applied in real-world scenarios enhances both learning and practical problem-solving abilities.
By mastering these key areas, you will be prepared to tackle challenges with confidence and precision, whether in academic settings or in various industries.
Mastering Advanced Mathematical Principles for Success
This section is dedicated to providing a deep understanding of the essential concepts and techniques required to excel in complex problem-solving scenarios. By focusing on key theorems, strategic approaches, and methods for improving your performance, you will be well-equipped to handle various challenges effectively.
Core Theorems and Important Concepts

Grasping fundamental principles is critical when dealing with intricate exercises. These core concepts serve as the foundation for solving problems efficiently. Whether dealing with spatial relationships or calculating measurements, understanding these theorems will enhance your problem-solving abilities.
- Pythagoras’ Theorem: A vital relationship in right-angled shapes.
- Congruency Principles: Essential for proving equality in geometrical figures.
- Area and Volume Formulae: Used to calculate size and space in various objects.
Effective Problem-Solving Strategies
When tackling complex problems, it’s important to break down each challenge into smaller, more manageable parts. Recognizing patterns and applying the right techniques is key to reaching a solution quickly and accurately.
- Dissecting the Problem: Analyze the question thoroughly before jumping into calculations.
- Recognizing Patterns: Identifying trends or repeating elements simplifies the process.
- Double-Checking Results: Ensure calculations are correct to avoid errors.
By mastering these core skills, you’ll not only be prepared for exams but also equipped to apply these techniques in real-world situations.
Reviewing Key Questions and Enhancing Accuracy

In many assessments, certain types of questions appear more frequently. Being familiar with these can help you anticipate what’s coming and practice more effectively. Additionally, focusing on accuracy will ensure your solutions are reliable, boosting your confidence during evaluations.
Refining your technique through regular practice and understanding the structure of common questions will significantly enhance your performance.