In this section, we will explore the fundamental concepts surrounding shapes with four sides. Understanding their properties is essential for solving a wide range of problems that you might encounter in your studies. By breaking down their characteristics and relationships, you can gain a deeper understanding of how these figures function within different mathematical contexts.
Focusing on key principles will allow you to identify patterns and apply logical reasoning when faced with various challenges. Whether you are dealing with angles, side lengths, or specific properties that define these shapes, mastering the core aspects will set a solid foundation for more advanced topics.
Step-by-step methods will guide you through common problem types, helping you recognize when specific strategies should be applied. Gaining proficiency in working with four-sided shapes is not only beneficial for exams but also for everyday applications where spatial reasoning plays a role.
Understanding Four-Sided Figures
In this section, we will delve into the world of shapes that have four edges, exploring their various properties and relationships. These figures are prevalent in many aspects of mathematics and real-world applications, making it crucial to understand how they behave in different situations. By recognizing the key characteristics of these shapes, you can approach problems involving them with more confidence and clarity.
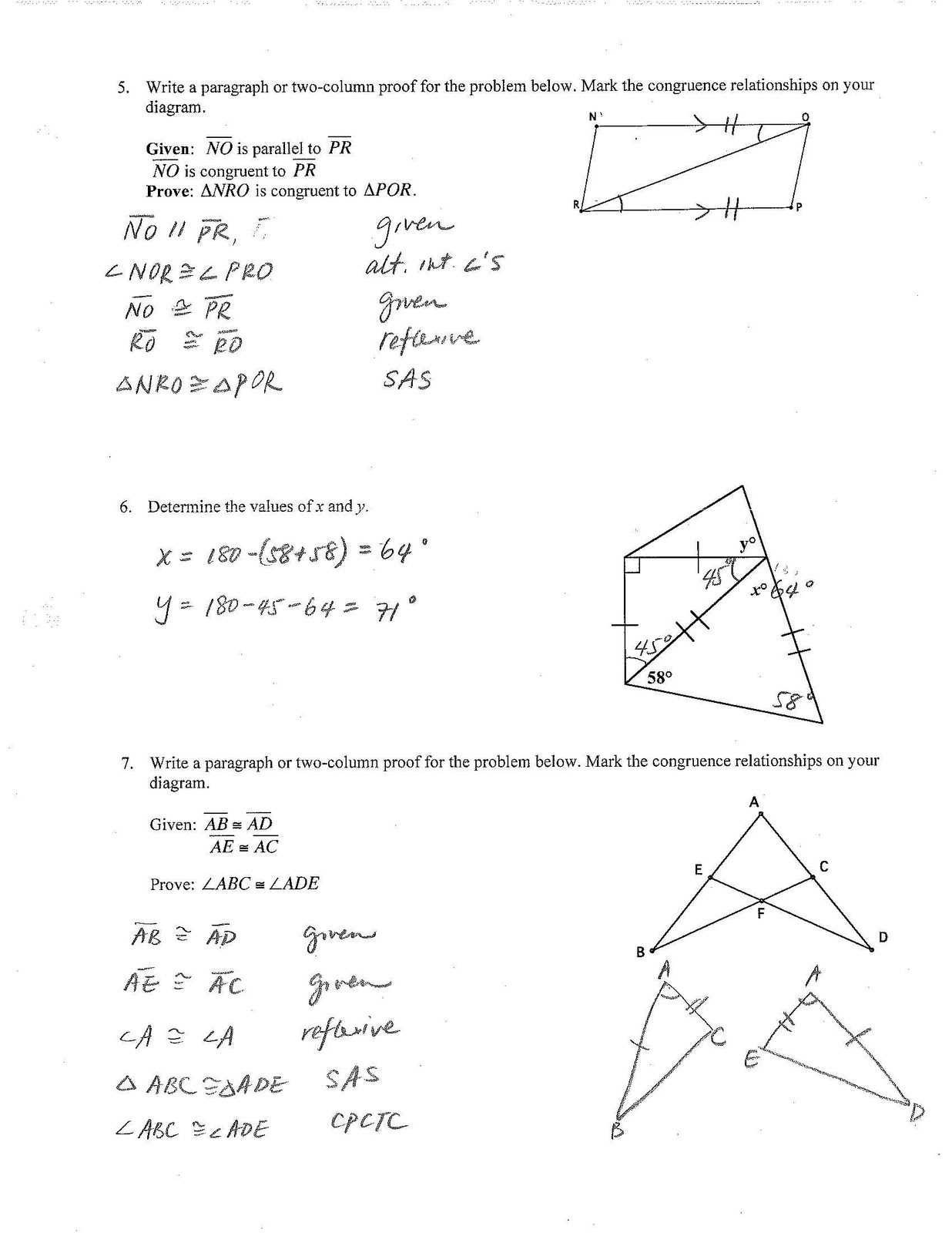
The focus is on identifying specific traits such as side lengths, angles, and symmetry. Each type of four-sided figure has its own set of rules that govern its structure, and understanding these rules is essential for solving related challenges. Whether dealing with equal sides, parallel lines, or specific angle measures, these concepts form the basis of more advanced problem-solving techniques.
As you progress, you will learn how to distinguish between different shapes based on their unique properties. This knowledge will help you tackle more complex scenarios by applying the right strategies and formulas. Developing a strong grasp of these principles will enhance your ability to solve related problems effectively and efficiently.
Key Properties of Four-Sided Figures
When studying shapes with four edges, it is essential to recognize the various characteristics that define their structure. These properties determine how such shapes interact with one another and how they can be manipulated in mathematical problems. By understanding these key features, you can better approach challenges that involve these figures.
Each figure in this category has unique traits, from equal side lengths to specific angle measures. Some figures exhibit symmetry, while others may have parallel sides or particular relationships between opposite angles. Knowing these aspects helps to identify the type of figure you are working with and select the appropriate methods for solving related problems.
| Property | Description |
|---|---|
| Side Lengths | The measurement of each edge. Some shapes may have equal sides, while others vary in length. |
| Angles | The sum of the interior angles is always 360 degrees, but the individual angles can differ depending on the figure. |
| Parallel Sides | Some figures have pairs of sides that are parallel to each other, affecting their symmetry and properties. |
| Symmetry | Certain shapes may have one or more lines of symmetry, meaning they can be folded along these lines to create identical halves. |
These basic properties form the foundation for solving problems involving these shapes, whether they are used in theoretical math or applied in real-world scenarios. By mastering these concepts, you can develop a deeper understanding of their behavior and characteristics.
How to Tackle Related Questions
When approaching problems that involve shapes with four sides, it’s important to follow a systematic approach. By breaking down the question into smaller, manageable parts, you can identify the key elements that need to be addressed. This method not only makes the problem-solving process more efficient but also reduces the likelihood of errors.
Start by carefully reading the question to understand what is being asked. Identify the known and unknown variables, and determine which properties of the figure are relevant. From there, follow a step-by-step process to apply the right strategies and formulas. Below are some key steps to keep in mind when tackling these problems:
- Identify the type of shape – Recognizing the figure’s characteristics (parallel sides, angle measures, side lengths) is crucial for applying the correct method.
- Use relevant formulas – Once you know the type of figure, select the appropriate formulas for calculating areas, perimeters, or angles.
- Draw diagrams – Sketching the shape can help visualize the relationships between the sides and angles, aiding in solving the problem more easily.
- Check for special properties – Look for any symmetry, parallel sides, or equal angles that might simplify the calculation or provide additional insights.
- Recheck the work – After solving the problem, verify your calculations to ensure accuracy and consistency with the known properties.
By following these steps, you will be able to approach any question confidently and with a methodical mindset. Whether it’s calculating missing sides or determining specific angles, breaking the problem into clear steps is the key to finding the solution.
Common Errors in Problem Solving
When working with shapes that have four sides, many individuals make mistakes due to misunderstandings or oversights of key concepts. These errors often stem from misinterpreting the problem, overlooking important details, or applying the wrong methods. Recognizing and addressing these common pitfalls will help improve your accuracy and confidence when solving related challenges.
Misunderstanding Properties
A frequent mistake occurs when the properties of the shape are not fully understood or misapplied. For example, confusing parallel sides with perpendicular ones or assuming that all angles are equal without checking can lead to incorrect results. It is essential to carefully review the figure’s characteristics before applying formulas or reasoning.
Calculation Mistakes
Another common error is in the calculations themselves. This could involve simple arithmetic errors or misapplying formulas. For instance, when calculating areas or perimeters, incorrect use of side lengths or angles can lead to wrong answers. Double-checking each calculation is key to avoiding this type of mistake. In some cases, reworking the problem from the beginning may reveal the source of the error.
Attention to detail and understanding the properties of the shape are crucial in preventing these mistakes. By recognizing these common errors and addressing them before they occur, you can significantly improve your problem-solving skills and reduce the likelihood of making errors in future exercises.
Step-by-Step Solutions for Practice Exercises
Working through practice exercises is one of the most effective ways to master problems involving four-sided figures. By breaking down each problem into smaller steps, you can develop a clear approach and identify any areas where you may need more practice. This section will guide you through the process of solving sample problems from start to finish.
Step 1: Understand the Problem

The first step is always to carefully read the problem to ensure you fully understand what is being asked. Identify all given information, such as side lengths, angle measures, or special properties of the figure. This will help you determine what needs to be calculated and what tools or formulas you will need to apply.
Step 2: Apply the Correct Method
Once you have a clear understanding of the problem, the next step is to choose the appropriate method for solving it. This could involve using formulas for area, perimeter, or calculating missing angles. For example, if the problem requires finding the perimeter, you would add up the lengths of all sides. If it involves angles, you might need to apply angle sum rules or properties of parallel lines.
As you go through each practice exercise, make sure to write out every step clearly and double-check your work. This will help you stay organized and avoid making simple errors that can lead to incorrect results. By repeating this process, you’ll gradually improve your ability to solve these types of problems efficiently and accurately.
Using Diagrams to Visualize Shapes
Diagrams are essential tools for understanding the relationships between different components of a figure. By visualizing shapes, you can gain a clearer understanding of their properties and how various elements, such as sides and angles, interact. Drawing accurate diagrams not only helps in solving problems but also enhances your ability to recognize patterns and apply the right strategies.
When faced with a problem involving four-sided figures, creating a diagram allows you to visually represent the given information. This can help you easily identify important features such as parallel sides, angles, or symmetry, which can be crucial for solving the problem. Additionally, diagrams make it easier to perform calculations like determining side lengths or finding missing angles by clearly showing the relationships between different parts of the shape.
By practicing drawing precise diagrams, you will improve both your understanding of shapes and your ability to solve problems more effectively. Whenever you encounter a challenging question, take the time to sketch the shape and label the known information–this simple step can often make all the difference in arriving at the correct solution.
Tips for Mastering Geometry Concepts
To excel in solving problems involving four-sided figures, it is essential to grasp the key principles and improve your problem-solving techniques. Developing a strong foundation in understanding shape properties, measurement rules, and calculation strategies will boost both your confidence and accuracy. With the right approach, mastering these concepts becomes an achievable goal.
Start by familiarizing yourself with basic definitions and properties. Knowing the different types of shapes, their characteristics, and how to apply relevant formulas is crucial. Regular practice is equally important–solving a variety of problems helps reinforce your understanding and sharpens your ability to tackle different scenarios. As you work through problems, be mindful of common mistakes and learn how to avoid them by paying close attention to the details in each question.
Another effective strategy is to visualize the problem. Drawing diagrams and labeling important information can often make the solution process easier and more straightforward. By connecting abstract concepts to concrete visual representations, you gain a deeper understanding of how each element of the shape functions within the problem.