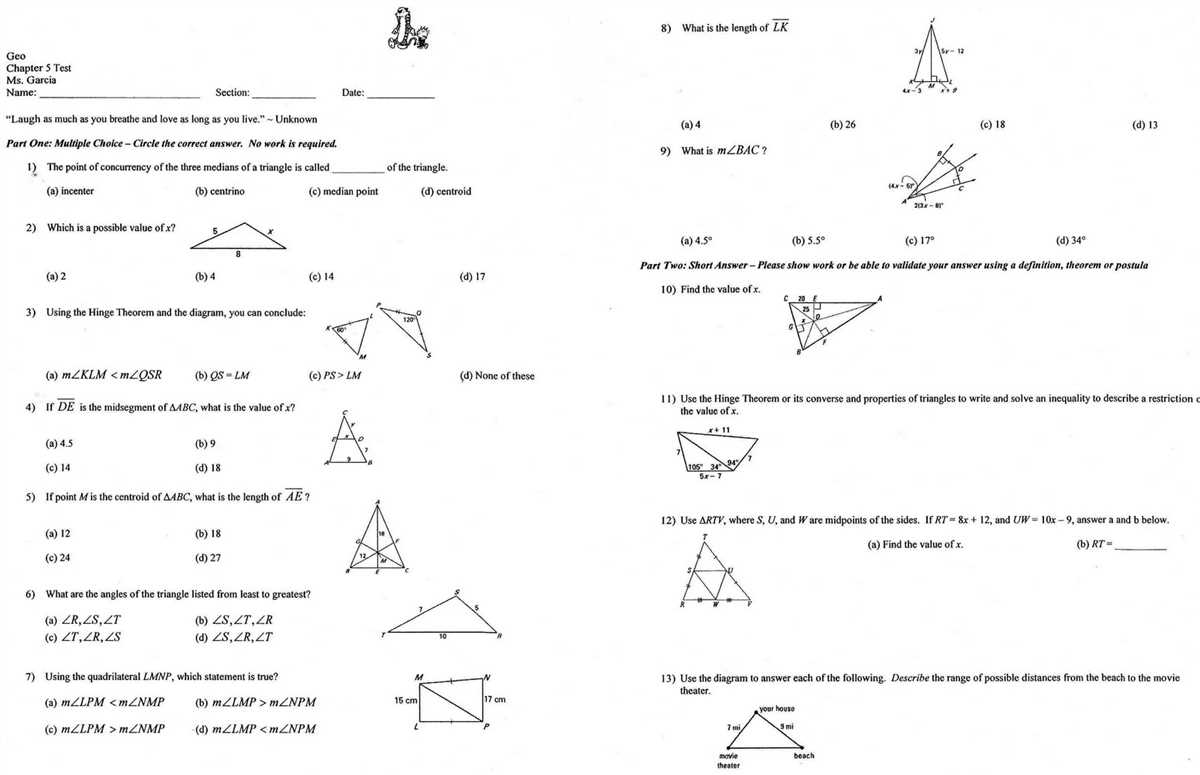
Geometry Chapter 9 covers various topics related to triangles and their properties. This chapter is crucial as it serves as a review for the concepts learned throughout the year. In this article, we will provide the answers to the review questions from Chapter 9, allowing students to check their understanding and strengthen their knowledge of geometry.
The review questions cover a range of topics, including triangle congruence, special triangles, and triangle inequalities. By going through these questions and checking the answers, students can assess their grasp of these concepts and identify any areas where they may need additional practice or review.
Understanding the properties of triangles is fundamental in geometry, as triangles are one of the most common shapes encountered. By reviewing the answers to the Chapter 9 questions, students can solidify their understanding of these properties and enhance their problem-solving skills.
Geometry Chapter 9 Review Answers
In geometry, Chapter 9 focuses on the properties and relationships of circles. This review provides answers to various questions related to the topics covered in this chapter.
1. What is the equation for the circumference of a circle?
The equation for the circumference of a circle is C = 2πr, where C represents the circumference and r represents the radius of the circle.
2. How is the area of a circle calculated?
The area of a circle is calculated using the equation A = πr², where A represents the area and r represents the radius of the circle.
3. What is the relationship between the radius, diameter, and circumference of a circle?
The radius and diameter of a circle are related by the equation d = 2r, where d represents the diameter and r represents the radius. The circumference of a circle is also related to the diameter by the equation C = πd.
4. How do you find the length of an arc?
To find the length of an arc, you can use the formula L = (θ/360) × 2πr, where L represents the length of the arc, θ represents the central angle in degrees, and r represents the radius of the circle.
5. What is the relationship between intercepted arcs and their corresponding central angles?
In a circle, intercepted arcs and their corresponding central angles are congruent, meaning they have the same measure.
6. How do you find the area of a sector?
The area of a sector can be found using the formula A = (θ/360) × πr², where A represents the area of the sector, θ represents the central angle in degrees, and r represents the radius of the circle.
7. What is the relationship between a tangent and a radius of a circle?
In a circle, a tangent line is perpendicular to the radius drawn to the point of tangency. This means that the tangent line intersects the circle at exactly one point.
8. How do you find the length of a chord?
To find the length of a chord, you can use the formula 2r × sin(θ/2), where r represents the radius of the circle and θ represents the central angle in radians.
9. What is the relationship between the lengths of two intersecting chords in a circle?
In a circle, if two chords intersect inside the circle, the product of the lengths of the segments of one chord is equal to the product of the lengths of the segments of the other chord.
10. How do you find the length of a tangent segment?
The length of a tangent segment can be found using the formula √((r₁ × r₂)), where r₁ and r₂ represent the radii of two circles that are externally tangent to each other.
In conclusion, this review of Geometry Chapter 9 provides answers to important questions related to the properties and relationships of circles. Understanding these concepts is crucial in solving problems and applying geometric principles involving circles.
Overview of Geometry Chapter 9
In Chapter 9 of our Geometry textbook, we dive into the fascinating world of circles, their properties, and various concepts related to them. This chapter serves as a comprehensive review of the key principles and theorems associated with circles, enabling students to deepen their understanding and master the application of these concepts in problem-solving scenarios.
The chapter begins by introducing the basic elements of a circle, such as the center, radius, and diameter. Students learn how to calculate the circumference and area of a circle using the appropriate formulas. Additionally, they explore the concept of a chord, which is a line segment connecting two points on the circle, and the relationships between chords and other geometric elements.
One of the central topics covered in this chapter is the theorem known as the Inscribed Angle Theorem. Students learn how to determine the measure of an inscribed angle using various techniques and properties, such as the relationship between inscribed angles and central angles, as well as the intercepted arcs.
The chapter also focuses on the relationship between tangent lines and circles. Students discover the properties of tangent lines and how they intersect with circles at a single point, called the point of tangency. They learn to identify tangent lines and use their properties to solve problems involving circles in real-life situations.
Lastly, the chapter covers the concept of a secant line, which intersects a circle at two points, and the relationships between secant lines and other geometric elements. Students explore how to calculate the length of secant segments, as well as understand the relationship between secants, tangents, and chords.
In conclusion, Chapter 9 provides a comprehensive review of the properties and concepts related to circles, preparing students to apply their knowledge in solving various geometry problems. By mastering the material covered in this chapter, students will gain a solid foundation in circle geometry, paving the way for more advanced topics in future chapters.
Key Concepts and Formulas
In this review of Geometry Chapter 9, we will cover some key concepts and formulas that are essential for understanding and solving problems related to the topic. These concepts and formulas are used to calculate measurements and properties of various geometric shapes such as circles, polygons, and triangles.
Circle Formulas:
- The circumference of a circle is calculated using the formula: C = πd, where C is the circumference and d is the diameter.
- The area of a circle is calculated using the formula: A = πr^2, where A is the area and r is the radius.
Polygon Formulas:
- The perimeter of a polygon is calculated by adding up the lengths of all its sides.
- The area of a regular polygon is calculated using the formula: A = ½ × ap, where A is the area, a is the apothem, and p is the perimeter.
Triangle Formulas:
- The area of a triangle is calculated using the formula: A = ½bh, where A is the area, b is the base, and h is the height.
- The Pythagorean Theorem is used to find the length of a missing side in a right triangle: a^2 + b^2 = c^2, where a and b are the lengths of the two legs and c is the length of the hypotenuse.
These are just a few of the key concepts and formulas discussed in Geometry Chapter 9. By understanding and applying these formulas, you will be able to solve a variety of problems related to circles, polygons, and triangles. Practicing with different examples and exercises will help reinforce your understanding of these concepts and improve your problem-solving skills in geometry.
Types of Quadrilaterals
In geometry, a quadrilateral is a polygon with four sides. There are several types of quadrilaterals, each with its own unique properties and characteristics.
1. Square: A square is a quadrilateral with all four sides of equal length and all four angles at 90 degrees. It is a regular polygon and has both rotational and reflectional symmetry.
2. Rectangle: A rectangle is a quadrilateral with opposite sides of equal length and all four angles at 90 degrees. It has rotational symmetry but not reflectional symmetry. Its diagonals are also equal in length.
3. Rhombus: A rhombus is a quadrilateral with all four sides of equal length. Its opposite angles are equal, but its opposite sides are not necessarily parallel. It has both rotational and reflectional symmetry.
4. Parallelogram: A parallelogram is a quadrilateral with opposite sides that are both parallel and equal in length. Its opposite angles are also equal. It does not have any symmetry.
5. Trapezoid: A trapezoid is a quadrilateral with one pair of opposite sides that are parallel. Its other pair of sides are not parallel. It does not have any symmetry.
6. Kite: A kite is a quadrilateral with two pairs of adjacent sides that are equal in length. Its diagonals intersect at a right angle. It does not have any symmetry.
7. Quadrilateral: A quadrilateral is a general term for any polygon with four sides. It includes all types of quadrilaterals mentioned above, as well as irregular quadrilaterals.
- A square is a special type of quadrilateral that has all sides and angles equal.
- A rectangle is a type of quadrilateral that has equal opposite sides and angles.
- A rhombus is a quadrilateral with equal sides but opposite angles may not be equal.
- A parallelogram is a quadrilateral with opposite sides that are parallel and equal.
Properties of Quadrilaterals
Quadrilaterals are four-sided polygons that have unique properties and characteristics. Understanding these properties can help us identify and classify different types of quadrilaterals.
1. Parallelogram: A parallelogram is a quadrilateral with two pairs of parallel sides. This means that the opposite sides are parallel and congruent. Additionally, the opposite angles are congruent and the consecutive angles are supplementary (add up to 180 degrees).
2. Rectangle: A rectangle is a parallelogram with four right angles. This means that all angles in a rectangle are 90 degrees. The opposite sides of a rectangle are congruent, and the diagonals bisect each other.
3. Rhombus: A rhombus is a parallelogram with four congruent sides. This means that all sides in a rhombus are equal in length. The opposite angles are congruent, and the diagonals bisect each other at right angles.
4. Square: A square is a rectangle with four congruent sides. This means that all sides in a square are equal in length. Additionally, all angles in a square are 90 degrees. The diagonals of a square bisect each other at right angles.
5. Trapezoid: A trapezoid is a quadrilateral with one pair of parallel sides. The non-parallel sides are called legs, while the parallel sides are called bases. The angles formed by the bases and the legs can vary.
6. Kite: A kite is a quadrilateral with two pairs of adjacent congruent sides. This means that the consecutive sides in a kite are equal in length. The diagonals of a kite bisect each other at right angles.
- A parallelogram has two pairs of parallel sides.
- A rectangle has four right angles.
- A rhombus has four congruent sides.
- A square is a rectangle with four congruent sides.
- A trapezoid has one pair of parallel sides.
- A kite has two pairs of adjacent congruent sides.
Sample Problems and Solutions
Here are some sample problems from the Geometry chapter 9 review, along with their solutions:
Problem 1:
The measures of the angles of a triangle are in the ratio 3:4:5. Find the measure of each angle.
Solution:
Let the measures of the angles be 3x, 4x, and 5x. Since the sum of the measures of the angles of a triangle is 180 degrees, we have the equation 3x + 4x + 5x = 180. Solving this equation, we find x = 12. Therefore, the measures of the angles are 36 degrees, 48 degrees, and 60 degrees.
Problem 2:
Two angles of a triangle measure 45 degrees and 75 degrees. Find the measure of the third angle.
Solution:
Since the sum of the measures of the angles of a triangle is 180 degrees, we have the equation 45 + 75 + x = 180, where x is the measure of the third angle. Solving this equation, we find x = 60. Therefore, the measure of the third angle is 60 degrees.
Problem 3:
In triangle ABC, angle A measures 30 degrees and angle B measures 75 degrees. Find the measure of angle C.
Solution:
Since the sum of the measures of the angles of a triangle is 180 degrees, we have the equation 30 + 75 + x = 180, where x is the measure of angle C. Solving this equation, we find x = 75. Therefore, the measure of angle C is 75 degrees.
These sample problems should give you a good understanding of the concepts covered in Chapter 9 of the Geometry book. Remember to practice more problems to solidify your knowledge and prepare for the upcoming test or exam. Good luck!