
Preparing for your final exam can be a daunting task, especially when it comes to a subject like geometry. With its complex formulas and abstract concepts, it’s easy to feel overwhelmed. But fear not! This review answer key is here to guide you through the process, ensuring that you are well-prepared for your exam.
Geometry is the study of shapes, sizes, and properties of figures and spaces. It is an essential branch of mathematics that plays a crucial role in various fields such as architecture, engineering, and art. Throughout the semester, you have covered a wide range of topics, including angles, triangles, quadrilaterals, circles, and three-dimensional figures.
This review answer key is designed to help you practice and reinforce the concepts you have learned throughout the semester. It provides detailed explanations and step-by-step solutions to various problems that you are likely to encounter on your final exam. By working through these problems and carefully studying the explanations, you will gain a deeper understanding of the material.
Geometry Semester 1 Final Exam Review Answer Key
In the Geometry Semester 1 Final Exam Review, students will find the answer key to all the questions and exercises that were covered throughout the semester. The answer key is a valuable resource for students to check their understanding and correctness of their solutions. It provides a clear and concise explanation of how to solve each problem, along with the correct answer. The answer key can serve as a study guide for students to review and practice before taking the final exam.
The answer key includes solutions to various topics in geometry such as angles, lines, triangles, quadrilaterals, circles, and more. Each topic is organized in a logical manner, making it easy for students to navigate and find the specific question they need help with. The answer key is accompanied by detailed explanations and diagrams, allowing students to visualize the mathematical concepts and strengthen their understanding.
The Geometry Semester 1 Final Exam Review Answer Key can be used as a self-study tool or as a supplement to classroom instruction. It is designed to help students review and reinforce the concepts and skills they have learned in class. By using the answer key, students can identify areas where they need additional practice and focus their studying efforts accordingly.
Overall, the Geometry Semester 1 Final Exam Review Answer Key is an essential resource for students preparing for their final exam. It provides a comprehensive set of solutions and explanations to help students improve their problem-solving skills and achieve success in geometry.
About the Geometry Semester 1 Final Exam
The Geometry Semester 1 Final Exam is an assessment given at the end of the first semester of a geometry course. It tests students’ understanding of the topics covered throughout the semester and serves as a comprehensive review before moving on to the next semester. The exam consists of multiple-choice questions, short-answer questions, and problem-solving exercises.
This final exam is designed to assess students’ knowledge and skills in various geometry concepts, including lines, angles, triangles, quadrilaterals, circles, and transformations. Students are expected to demonstrate their ability to apply key principles and formulas to solve geometric problems. The exam also evaluates their proficiency in using geometric vocabulary and notation to communicate solutions effectively.
The Geometry Semester 1 Final Exam Answer Key provides the correct answers and explanations for each question on the exam. It allows students to check their work, identify areas of weakness, and learn from their mistakes. The answer key also serves as a valuable resource for teachers to review the exam and provide feedback to students.
Preparing for the Geometry Semester 1 Final Exam involves reviewing class notes, textbook materials, and practice problems. It is recommended that students create a study plan and allocate sufficient time to review each topic thoroughly. Working through sample exam questions and using the answer key to check their solutions can help students gain confidence and improve their performance on the actual exam.
In conclusion, the Geometry Semester 1 Final Exam is an important milestone in a geometry course, assessing students’ understanding and application of various geometric concepts. Utilizing the answer key as a study tool can help students prepare effectively and identify areas for improvement. With proper preparation and review, students can approach the final exam with confidence and achieve success.
Important Topics to Review
In order to prepare for the Geometry semester 1 final exam, it is important to review key topics that will be covered on the test. The following is a list of important concepts and skills that you should focus on:
- Angles and Lines: Review the properties of angles and lines, such as parallel lines, perpendicular lines, and angle relationships (e.g. vertical angles, alternate interior angles, corresponding angles).
- Triangles: Understand the properties of triangles, including the sum of the angles, the Pythagorean theorem, congruence criteria (e.g. SSS, SAS, ASA), and the properties of special triangles (e.g. equilateral, isosceles, right triangles).
- Quadrilaterals: Familiarize yourself with the properties of quadrilaterals, including parallelograms, rectangles, squares, rhombi, and trapezoids.
- Circles: Review the properties of circles, including the relationships between chords, arcs, tangents, and the formulas for finding the circumference and area of a circle.
- Polygons: Understand the properties of polygons, including regular polygons, interior and exterior angles, and the formulas for finding the perimeter and area of polygons.
- Coordinate Geometry: Be able to work with coordinates and equations of lines in the coordinate plane, including slope, distance, midpoint, parallel lines, and perpendicular lines.
- Transformational Geometry: Review transformations, such as translations, reflections, rotations, and dilations, and understand how they affect the shape and position of figures.
In addition to these specific topics, it is important to have a good understanding of basic geometric vocabulary and notation, as well as problem-solving strategies, such as proofs and geometric constructions. By reviewing these key topics and practicing with sample problems, you will be well-prepared for the Geometry semester 1 final exam.
Key Concepts and Formulas
1. Basic Geometric Definitions
Before diving into the formulas, it is important to understand some fundamental geometric definitions. These include:
- Points: Represented by a dot and have no size or dimension.
- Lines: Defined by two points and extend indefinitely in both directions.
- Line segments: Part of a line between two points. Can be measured.
- Angles: Formed by two rays with a common endpoint. Measured in degrees.
- Triangles: Polygons with three sides and three angles.
2. Perimeter and Area Formulas
The perimeter is the sum of all the lengths of the sides of a polygon. The formulas for the perimeter and area of common shapes are as follows:
- Rectangle: Perimeter = 2(length + width), Area = length × width
- Triangle: Perimeter = sum of all three side lengths, Area = 0.5(base × height)
- Circle: Perimeter (or circumference) = 2πr, Area = πr²
3. Pythagorean Theorem
The Pythagorean theorem is a fundamental formula in geometry that relates the sides of a right triangle. It states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. The formula can be written as:
a² + b² = c²
where:
- a and b represent the lengths of the two legs of the triangle
- c represents the length of the hypotenuse
4. Trigonometric Ratios
Trigonometric ratios are used to relate the angles of a right triangle to the ratios of its sides. The three primary ratios are:
- Sine (sin): The ratio of the length of the side opposite an angle to the length of the hypotenuse.
- Cosine (cos): The ratio of the length of the side adjacent to an angle to the length of the hypotenuse.
- Tangent (tan): The ratio of the length of the side opposite an angle to the length of the side adjacent to the angle.
These ratios can be calculated using the formulas:
- sin(θ) = opposite/hypotenuse
- cos(θ) = adjacent/hypotenuse
- tan(θ) = opposite/adjacent
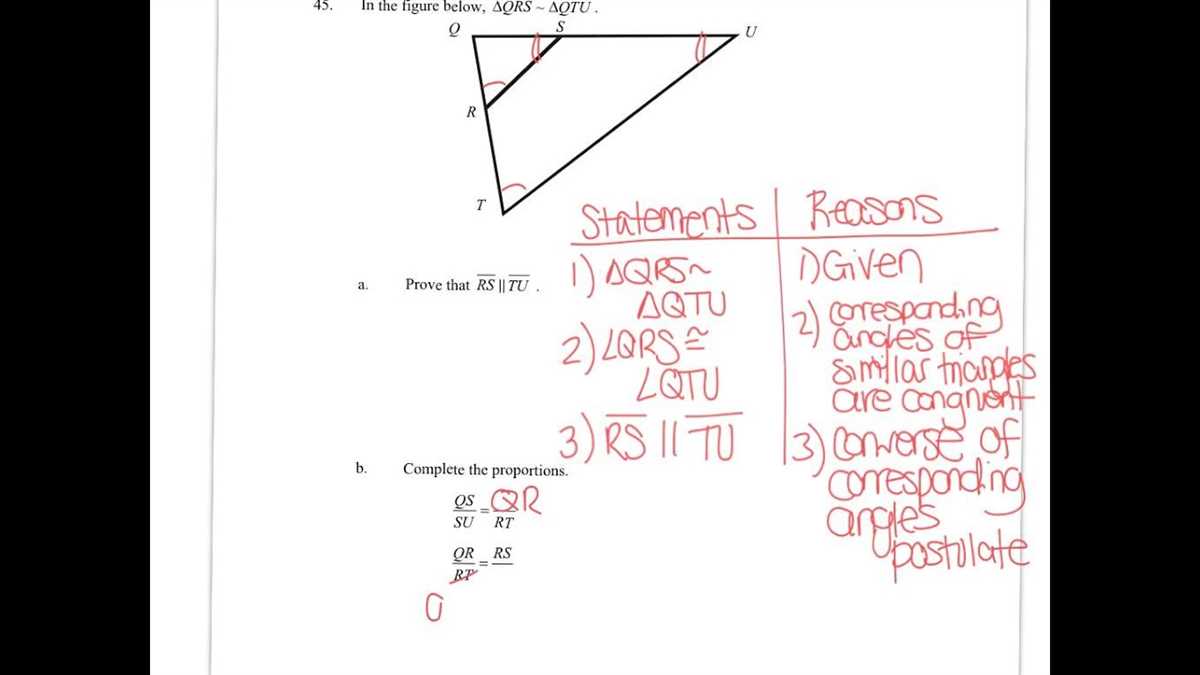
5. Similarity and Congruence
Understanding the concepts of similarity and congruence is crucial in geometry. Two figures are considered similar if they have the same shape but possibly different sizes. Congruent figures, on the other hand, have the same shape and size.
Sample Questions and Answers
Here are some sample questions and answers that can help you prepare for the Geometry semester 1 final exam:
1. Find the area of a rectangle with length 12 cm and width 7 cm.
To find the area of a rectangle, you multiply its length by its width. In this case, the length is 12 cm and the width is 7 cm. So, the area of the rectangle is 12 cm * 7 cm = 84 cm^2.
2. Determine the measure of angle ABC in the triangle below:

To determine the measure of angle ABC, we need to use the properties of triangles. The sum of the angles in a triangle is always 180 degrees. We can see that angle ACB is 60 degrees because it is an equilateral triangle. The two angles adjacent to it (angles ABC and BAC) must add up to 180 – 60 = 120 degrees. Therefore, angle ABC is 120/2 = 60 degrees.
3. Find the length of side AB in the right triangle below:
In a right triangle, the sum of the squares of the lengths of the legs (the sides adjacent to the right angle) is equal to the square of the length of the hypotenuse (the side opposite the right angle). In this case, the lengths of the legs are 3 cm and 4 cm. So, 3^2 + 4^2 = 9 + 16 = 25. The square root of 25 is 5. Therefore, the length of side AB is 5 cm.
4. Calculate the perimeter of the trapezoid with bases measuring 7 cm and 12 cm, and a height of 5 cm.
The perimeter of a trapezoid is the sum of the lengths of its four sides. In this case, the lengths of the bases are 7 cm and 12 cm, and the height is 5 cm. The two non-parallel sides are equal in length, so we need to calculate the length of one of them. Using the Pythagorean theorem, we can find that the length of one of the non-parallel sides is √(12^2 – 5^2) = √(144 – 25) = √119. Therefore, the perimeter of the trapezoid is 7 cm + 12 cm + 2 * √119 cm = 7 cm + 12 cm + 2√119 cm.
These are just a few examples of the types of questions you may encounter in the Geometry semester 1 final exam. It is important to study and practice different types of problems in order to become familiar with the concepts and strategies needed to solve them. Good luck!
Study Tips for Success
Preparing for a geometry final exam can be challenging, but with the right study strategies, you can ensure success. Here are some tips to help you effectively review and prepare:
1. Organize your notes:
Start by organizing your class notes and assignments. Create a clear and logical system to review concepts, theorems, and formulas. This will help you easily access and review specific topics during your study sessions.
2. Practice with examples:

Geometry involves a lot of problem solving, so practice is essential. Work through plenty of example problems from your textbook or past assignments. This will reinforce your understanding of concepts and help you develop problem-solving skills.
3. Collaborate with classmates:
Studying with classmates can be beneficial as it allows for discussions, exchange of ideas, and peer teaching. Form a study group and review concepts together. Explaining concepts to others can solidify your own understanding and provide different perspectives.
4. Utilize online resources:
Take advantage of online resources such as educational websites, tutorials, and practice quizzes. These resources can provide additional explanations, visual demonstrations, and extra practice problems to further enhance your understanding.
5. Review past exams:
Reviewing past exams or practice tests can give you a sense of the types of questions that may appear on your final exam. Pay attention to the areas where you struggled and focus on improving your understanding in those areas.
- 6. Seek help when needed:
- If you are struggling with specific concepts or topics, don’t hesitate to seek help from your teacher, classmates, or tutors. They can provide additional explanations and clarify any confusion you may have.
By following these tips and consistently dedicating time to study, you can approach your geometry final exam with confidence and increase your chances of success.
Final Thoughts
In conclusion, the Geometry semester 1 final exam review has covered a range of topics and concepts that students need to be familiar with in order to succeed on their final exam. Throughout this review, we have refreshed our knowledge on important geometric principles, such as angles, lines, and shapes. We have also practiced problem-solving skills by working through various types of geometry problems.
It is important to note that this review is not exhaustive and may not cover every possible question that could appear on the final exam. However, it provides a solid foundation and serves as a valuable resource for students to test their understanding of the material.
Remember to continue practicing and reviewing the material on your own, seeking help from your teacher or classmates when needed. Use additional resources, such as textbooks or online tutorials, to further enhance your understanding of the topics covered in this review.
Good luck with your final exam, and remember to stay confident in your abilities. With dedication and hard work, you will be well-prepared to tackle any geometry problem that comes your way.