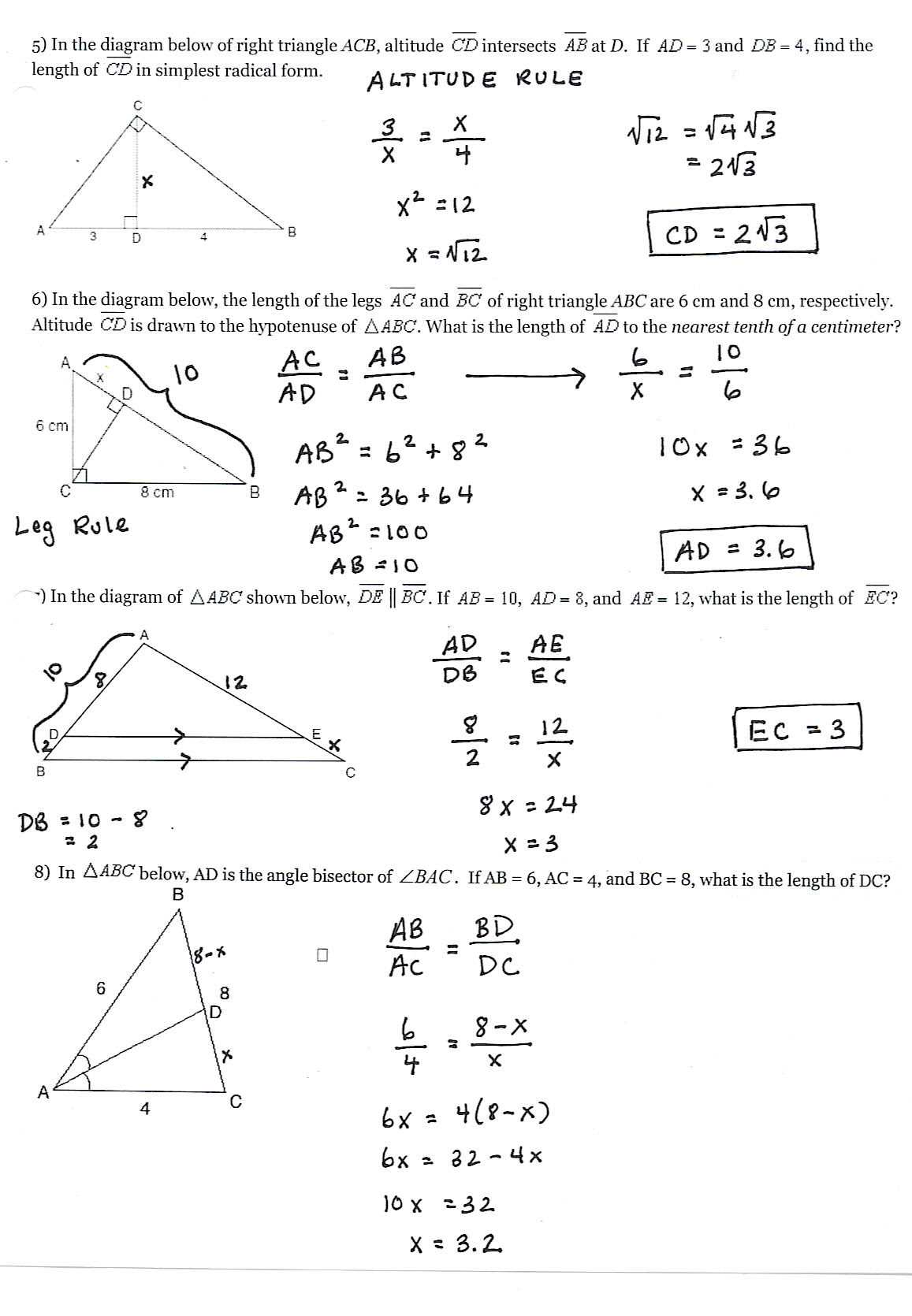
This section focuses on mastering key concepts and solving critical exercises. It aims to sharpen problem-solving skills and prepare you for complex challenges. By practicing various types of questions, you will develop a deeper understanding of essential theories and techniques.
Essential Concepts for Success
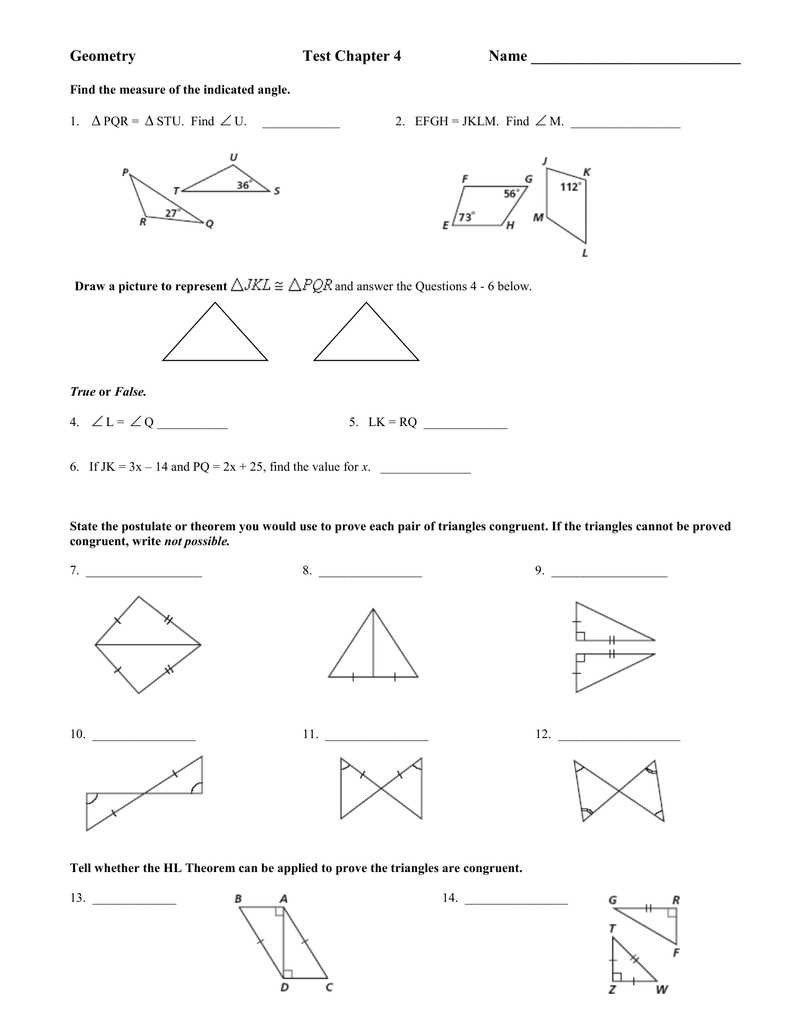
Before diving into problems, it is important to understand the core ideas. The foundation consists of principles and theorems that guide the solution process. Having a solid grasp of these ideas will help you approach each question with confidence and clarity.
Understanding Fundamental Theorems
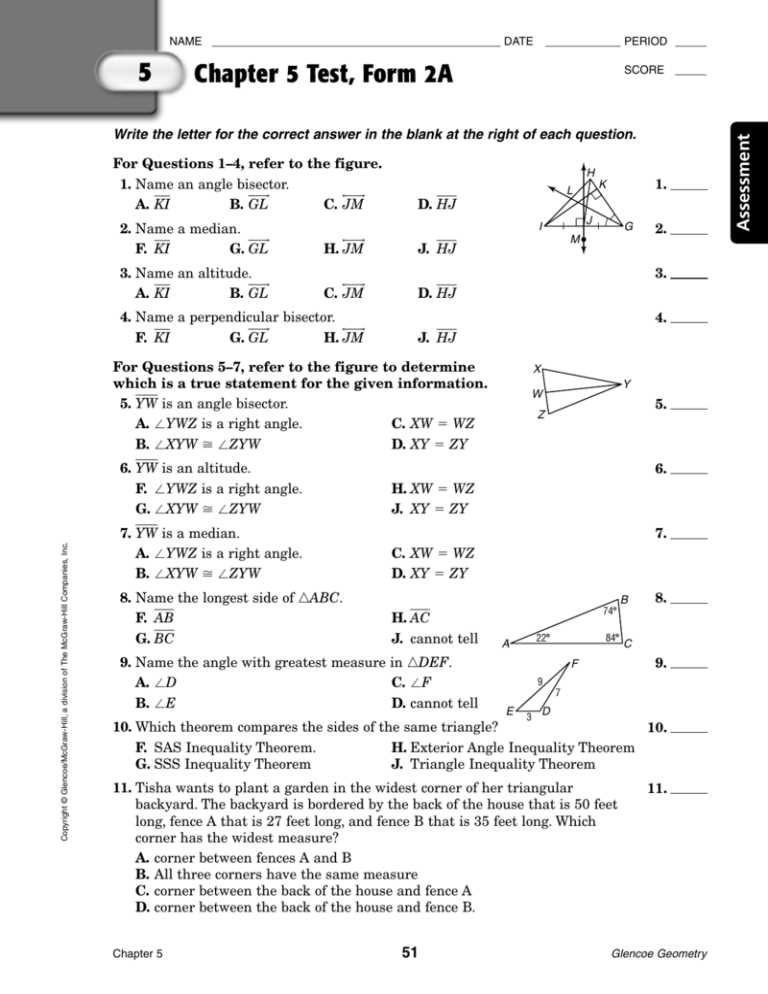
- Learn the properties that define different shapes.
- Explore relationships between angles and lines.
- Study how to apply various rules to find unknowns.
Practical Application of Theories
Once you are familiar with the concepts, applying them becomes the next step. Practice solving problems that involve various scenarios. This will allow you to recognize patterns and choose the most effective methods for each case.
Step-by-Step Problem Solving Approach
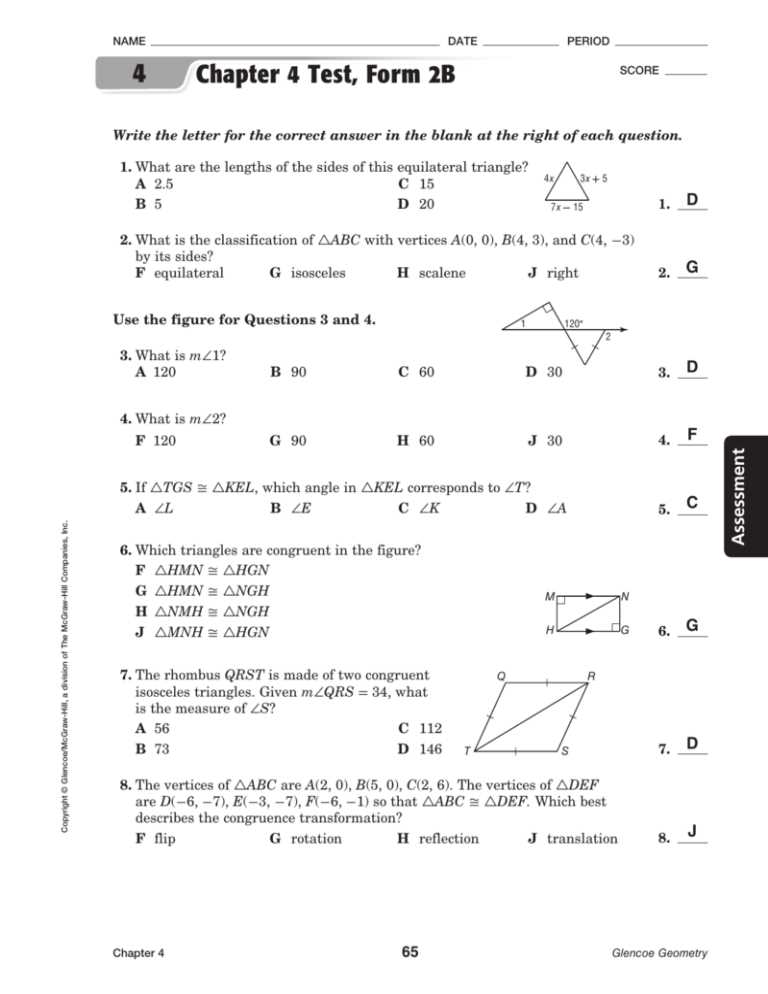
Breaking down each problem into smaller steps is a critical technique for success. Start by identifying the given information and determining what is required. Then, apply the appropriate rules to find a solution.
Example Problem Breakdown
- Identify the known variables and their relationships.
- Apply the relevant formulas to set up equations.
- Solve the equations and check the results for accuracy.
Avoiding Common Mistakes
Throughout this section, it’s easy to make small errors that could lead to incorrect answers. Be mindful of miscalculations or overlooked steps. Always double-check your work to ensure accuracy.
Practical Tips for Improvement
- Practice regularly with a variety of problems.
- Focus on understanding the reasoning behind each step.
- Review past problems to identify recurring patterns and techniques.
Review of Key Concepts and Problem Solving Strategies
This section provides an in-depth look into essential theories and methods required to solve complex exercises. Understanding the main principles, applying them to specific tasks, and avoiding common mistakes will enhance your ability to tackle any problem effectively. Consistent practice with a variety of scenarios will lead to mastery.
First, grasp the fundamental concepts that form the foundation of the exercises. Knowing these principles will allow you to approach each problem with the right tools and strategies. Recognizing the connections between different elements will make solving tasks more intuitive and efficient.
Once you understand the key concepts, the next step is applying them through a structured approach. Break each task into manageable steps, identifying the given information and required outcomes. Use the appropriate formulas and techniques for each situation, working through problems methodically to ensure accuracy.
Throughout this process, it’s important to be aware of common errors. Mistakes often occur when the rules are applied incorrectly or when crucial details are overlooked. Pay close attention to the specifics of each question and avoid rushing through calculations. Careful review and attention to detail can prevent simple missteps.
In order to perform well, you need to know how to approach each question systematically. Start by analyzing what is being asked and consider the best method to solve it. Prioritize understanding the reasoning behind each step, which will help you adapt your approach to different types of problems.
Additionally, preparation is key to success. Regular practice with a range of exercises will help build confidence and improve speed. Make sure to review mistakes to understand where improvement is needed and focus on areas that are challenging.
Finally, practice as much as possible to ensure mastery. Work through multiple problems, checking each solution for accuracy. The more you practice, the more familiar you will become with the techniques, making it easier to approach new problems with ease and certainty.