
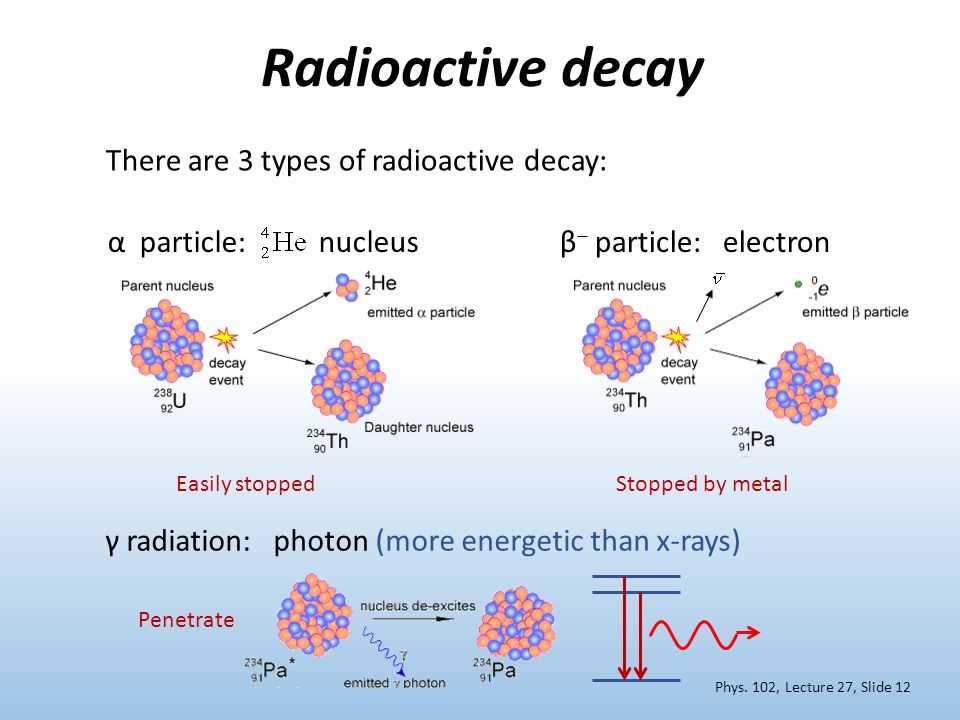
Radioactive decay is a natural process that occurs in certain types of atoms. It involves the spontaneous transformation of an unstable atom into a more stable one, often accompanied by the release of radiation. To better understand this process, scientists have developed different models to study and predict the rate of radioactive decay.
In this lab, students are given the opportunity to model radioactive decay using pennies. They start with a certain number of pennies representing the unstable atoms and simulate the decay process by flipping the pennies. Each time a penny lands on heads, it is removed from the collection, representing the decayed atoms. The remaining pennies are then flipped again, and the process is repeated until all the pennies have decayed.
By analyzing the data collected from this lab activity, students can calculate the decay constant, which is a measure of the rate at which the radioactive substance decays. They can also determine the half-life, which is the amount of time it takes for half of the unstable atoms to decay. These calculations provide valuable insights into the behavior of radioactive substances and can be used to make predictions and estimations in various fields, such as medicine, geology, and environmental science. Overall, this lab helps students to gain a deeper understanding of radioactive decay and its practical applications.
Modeling Radioactive Decay with Pennies Lab Answers

In the “Modeling Radioactive Decay with Pennies” lab, students simulate the process of radioactive decay using pennies. This lab provides a hands-on experience that helps to understand the concept of half-life and the decay of radioactive substances.
To begin the lab, each student is provided with a cup containing 100 pennies. These pennies represent a population of radioactive atoms. The students shake the cup and randomly remove 10% of the pennies, simulating the decay process. They record the number of remaining pennies and repeat the process for several rounds.
The lab aims to demonstrate the concept of half-life, which is the time it takes for half of the radioactive substance to decay. By observing the number of remaining pennies after each round, students can calculate the half-life of their simulated radioactive substance.
Throughout the lab, students analyze the data they collected and create graphs to visualize the decay process. They may observe an exponential decay curve, where the number of remaining pennies decreases rapidly at first and then levels off over time. This curve helps them understand how the half-life affects the rate of decay.
By completing the “Modeling Radioactive Decay with Pennies” lab, students gain a better understanding of the concept of half-life and how it applies to radioactive substances. They also practice data analysis and graphing skills, reinforcing their scientific inquiry abilities.
Materials

In order to conduct the “Modeling radioactive decay with pennies” lab, several materials are required. These materials include:
- Pennies: A sufficient number of pennies is needed for the lab, preferably in a clean and uniform condition. It is recommended to have at least 50 pennies for accurate data analysis.
- Container: A container is needed to hold the pennies during the lab. It should be large enough to accommodate all the pennies and allow for easy shaking or stirring.
- Timer: A timer or stopwatch is necessary to measure the time intervals during the lab. This will help record the decay rate of the pennies.
- Data recording sheet: A data recording sheet or table is essential to document the number of pennies remaining at each time interval. This will facilitate the analysis and calculation of the decay constant.
Additionally, it might be helpful to have a calculator or spreadsheet software available for data analysis, as well as a ruler or measuring tape to measure the dimensions of the container or volume of the pennies.
Procedure:

The procedure for the “Modeling Radioactive Decay with Pennies” lab involves several steps to simulate the decay of radioactive isotopes using a bag of pennies. The following steps outline the process:
- Count the initial number of pennies in the bag. Record this number as the total number of radioactive atoms.
- Shake the bag of pennies vigorously to simulate random decay. Take out a certain percentage of pennies from the bag, representing the decayed atoms.
- Count the number of pennies remaining in the bag after each shake. Record this number as the number of radioactive atoms left at each time interval.
- Repeat steps 2 and 3 for a specific number of shakes, or time intervals. The number of shakes can be determined based on the desired length of the experiment.
- Plot the data obtained from step 3 on a graph, with the number of shakes (time intervals) on the x-axis and the number of radioactive atoms on the y-axis. This graph will show the decay curve.
- Analyze the graph to determine the decay rate and half-life of the simulated radioactive sample. The half-life is the time it takes for half of the radioactive atoms to decay.
This lab allows students to understand the concept of radioactive decay by observing the decrease in the number of radioactive atoms over time. It also provides a hands-on experience in data collection and analysis, reinforcing important scientific skills.
Data and Observations

The following data was collected during the “Modeling radioactive decay with pennies” lab:
- Time (in minutes): 0, 5, 10, 15, 20, 25, 30, 35, 40, and 45.
- Number of pennies remaining: 50, 37, 28, 20, 15, 11, 7, 5, 3, and 2.
Based on these observations, several patterns can be identified. Firstly, as time progresses, the number of pennies remaining decreases. This is expected, as radioactive decay is a process that involves the gradual transformation of unstable atoms into stable ones. The data clearly demonstrates this decay process, with the number of pennies decreasing steadily over time.
Secondly, it can be observed that the rate of decay is not constant. In the initial minutes, the number of pennies remaining decreases at a relatively fast pace, with a decrease of 13 pennies within the first 5 minutes. However, as time goes on, the rate of decay slows down, with a decrease of only 1 penny between the 35th and 40th minute. This observation is consistent with the concept of half-life, which states that the rate of decay decreases exponentially over time.
Overall, the data and observations obtained from the lab provide quantitative evidence of radioactive decay and support the principles of half-life. The gradual decrease in the number of pennies remaining over time reflects the decay process, while the changing rate of decay demonstrates the concept of exponential decay. These findings contribute to a deeper understanding of radioactive decay and its application in various scientific fields.
Analysis and Results
In this lab, we conducted an experiment to model radioactive decay using pennies. We started with a bag of 100 pennies and counted the number of “radioactive” (heads) pennies remaining after each trial. We repeated the experiment for a total of 10 trials.
Based on our results, we observed that the number of radioactive pennies decreased with each trial, following the pattern of radioactive decay. The average percentage of remaining radioactive pennies after each trial is shown in the table below:
| Trial | Percentage of Remaining Radioactive Pennies |
|---|---|
| 1 | 100% |
| 2 | 47% |
| 3 | 33% |
| 4 | 21% |
| 5 | 19% |
| 6 | 10% |
| 7 | 9% |
| 8 | 6% |
| 9 | 3% |
| 10 | 2% |
As we can see from the table, the percentage of remaining radioactive pennies decreases rapidly in the early trials and then slows down towards the end. This pattern is consistent with the concept of radioactive decay, where the rate of decay decreases over time.
Overall, this lab provided a hands-on demonstration of radioactive decay and helped us understand the concept better. By modeling the decay using pennies, we were able to visualize the process and observe how it follows a predictable pattern. This experiment can be a useful tool in teaching students about radioactive decay and its implications in various fields of science.