
If you’re a student or just someone who loves math, you’ve probably encountered calculus at some point in your academic journey. Calculus is a branch of mathematics that deals with rates of change and accumulation of quantities, and it’s often considered one of the most challenging subjects to master. However, with the right guidance and resources, you can conquer calculus and find answers to all those perplexing problems.
In this article, we’ll explore different ways to approach calculus problems and find the answers you need. Whether you’re struggling with derivatives, integrals, or optimization, we’ll provide you with tips, techniques, and step-by-step solutions to help you navigate the intricacies of calculus. So, get ready to conquer your calculus woes and unlock the secrets of this fascinating branch of mathematics.
From finding the rate at which a quantity is changing to determining the area under a curve, calculus offers a powerful set of tools for solving real-world problems. Understanding the fundamental concepts and applying the appropriate techniques is crucial to finding accurate answers. So, whether you’re studying for an exam, working on a project, or simply want to explore the beauty of mathematics, our comprehensive guide will equip you with the knowledge and skills necessary to tackle calculus problems head-on.
What is calculus and why is it important?

Calculus is a branch of mathematics that deals with change and motion. It focuses on studying and analyzing how things change over time, as well as how to measure and model these changes. It is an essential tool in various fields such as physics, engineering, economics, and computer science, as it provides a framework for understanding and solving complex problems.
Calculus is divided into two main branches: differential calculus and integral calculus. Differential calculus focuses on studying instantaneous rates of change, while integral calculus focuses on finding the accumulation of quantities over time. Together, these branches form a powerful toolset for analyzing a wide range of phenomena.
Importance of calculus:
- Understanding motion and change: Calculus allows us to describe and analyze the motion and change of objects, whether it’s the trajectory of a projectile, the growth of a population, or the flow of fluids. By studying derivatives and integrals, we can gain insights into how things behave and predict their future behavior.
- Optimization and decision-making: Calculus plays a crucial role in optimization, which involves finding the best possible solution among many alternatives. Whether it’s maximizing profits, minimizing costs, or optimizing physical processes, calculus provides the tools to analyze and make informed decisions based on mathematical models.
- Understanding complex systems: Many real-world phenomena and systems are inherently complex, involving multiple variables and interactions. Calculus enables us to model and analyze these systems, making it possible to understand their behavior, identify patterns, and make predictions. This is crucial in fields like physics, economics, and engineering.
- Advancements in technology: Calculus is the foundation for many scientific and technological advancements. It underpins various fields of study, including computer science, artificial intelligence, and data analysis. Without calculus, we wouldn’t have the sophisticated algorithms and models that drive modern technologies.
In conclusion, calculus is an essential branch of mathematics that enables us to study and understand the dynamics of change and motion. Its importance extends beyond the field of mathematics, as it provides valuable tools for solving real-world problems, optimizing processes, and making informed decisions. Therefore, a solid understanding of calculus is crucial for any student or professional pursuing a career in STEM or related disciplines.
The basics of calculus: derivatives and limits
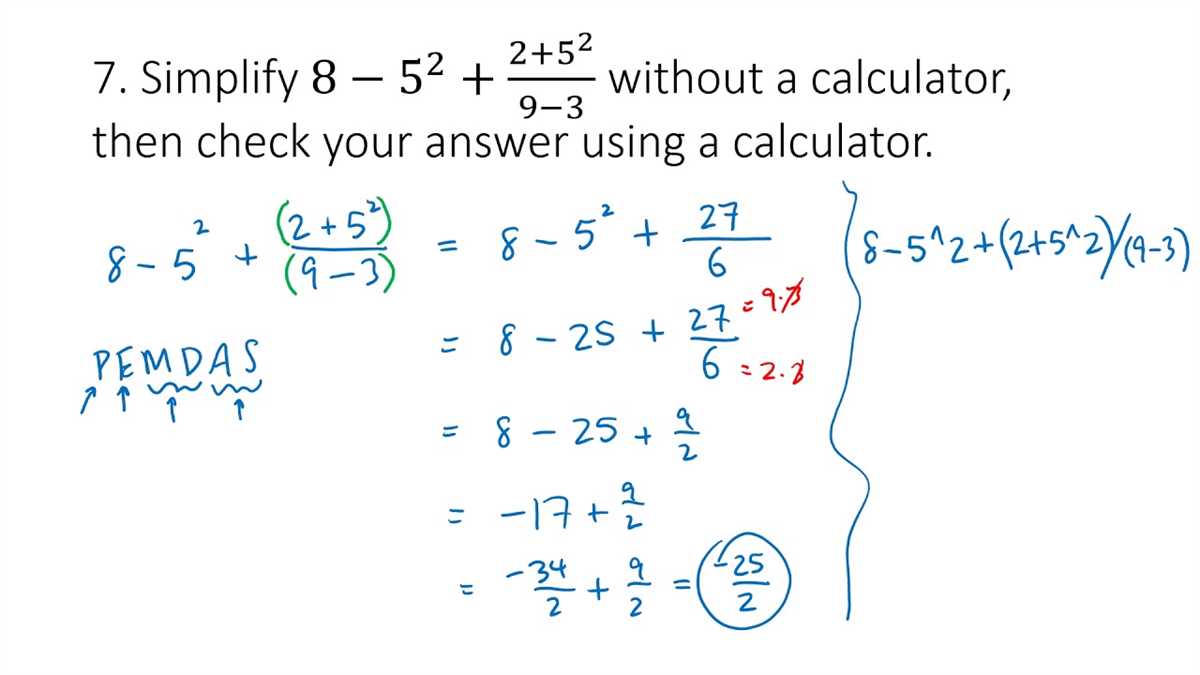
Calculus is a branch of mathematics that deals with the studies of change and motion. It is divided into two main concepts: derivatives and limits. Derivatives are used to measure the rate of change of a function at a particular point, while limits describe the behavior of a function as it approaches a certain value.
Derivatives are an essential concept in calculus. They allow us to calculate the instantaneous rate of change of a function at a given point. Derivatives are represented as the slope of a tangent line to a curve at a specific point. They help us determine how a function is changing at any specific point along its curve. The derivative of a function is denoted using the prime notation (‘) or by using the symbol dy/dx.
Limits are used to describe the behavior of a function as its input approaches a specific value. They enable us to understand the behavior of a function at infinity or when approaching a certain point. Limits are represented by two key parts: the input value and the value the function approaches as the input gets closer and closer. A limit can exist or not exist depending on whether or not the function approaches a specific value as the input approaches a certain point.
In conclusion, the basics of calculus revolve around derivatives and limits. Derivatives help us measure the rate of change of a function at a specific point, while limits describe the behavior of a function as it approaches a certain value. These concepts are fundamental in understanding the fundamental principles of calculus and are used in various applications in mathematics, physics, engineering, and other fields.
Understanding integration and its applications
Integration is an essential concept in calculus that allows us to find the area under curves and solve a wide range of problems in various fields of science and engineering. It involves the process of calculating the integral of a function, which represents the cumulative effect of the function over a given interval. Integration is closely related to differentiation, as they are inverse operations.
Integration has numerous applications in various fields. For example, in physics, integration is used to calculate the work done by a force on an object, the distance traveled by an object with varying velocity, and the electric field around a charged particle. In economics, integration is used to determine the total revenue or profit generated by a business, the marginal cost of production, and to model supply and demand curves.
One of the most important applications of integration is in finding the area under curves. This is useful in calculating the area of irregular shapes, such as the area of land or the volume of an irregularly shaped object. Integration offers a precise and systematic method for finding these areas by splitting them into infinitesimally small elements and summing them up.
Integration is also used in solving differential equations, which are equations that involve derivatives. By integrating both sides of the equation, we can find an equation that relates the original variables. This allows us to study and understand various phenomena, such as population growth, radioactive decay, and fluid flow.
In conclusion, integration is a fundamental concept in calculus that allows us to find areas, solve differential equations, and model various real-world phenomena. Its applications extend to a wide range of fields, including physics, economics, and engineering. Understanding integration is essential for anyone seeking to delve deeper into the world of mathematics and its practical applications.
Common problems in calculus and how to solve them
Calculus can be a challenging subject for many students, but with proper understanding and practice, you can overcome common problems that arise while studying it. Here are some common problems in calculus and strategies to solve them:
1. Difficulty understanding key concepts
One of the main challenges in calculus is grasping the fundamental concepts. Many students find it hard to understand the concept of limits, derivatives, and integrals. To overcome this problem, it is important to break down complex ideas into simpler components and relate them to real-world examples. Visual aids, such as graphs and diagrams, can also help in understanding these concepts better.
2. Trouble with solving problems step by step
Calculus often involves solving problems step by step, and it can be overwhelming for some students to keep track of all the calculations. To solve this problem, it is advisable to approach problems systematically and use a structured approach. Break down the problem into smaller parts, identify the necessary formulas or concepts to use, and then proceed with the calculations step by step. Practicing regularly and seeking help from teachers or peers can also improve problem-solving skills.
3. Lack of algebraic skills
Calculus heavily relies on algebraic manipulation and solving equations. If you struggle with algebra, it can make calculus more challenging. To improve your algebraic skills, review and practice basic algebraic operations, such as factoring, simplifying expressions, and solving equations. Understanding algebraic principles will make it easier to work through calculus problems.
4. Misinterpreting word problems
Word problems in calculus can be tricky, as they require translating the given information into mathematical equations. Misinterpreting the problem can lead to incorrect calculations and wrong answers. To avoid this, it is essential to read the problem carefully, identify the given information, and define the variables before setting up the equations. Practice solving various types of word problems to improve your problem-solving skills.
By addressing these common problems in calculus and applying the suggested strategies, you can enhance your understanding and proficiency in this field of mathematics. Remember to practice regularly, seek help when needed, and have a positive mindset towards learning calculus.
Advanced topics in calculus: differential equations and multivariable calculus
Differential equations and multivariable calculus are advanced topics in mathematics that build upon the fundamental concepts of calculus. These subjects delve into more complex and nuanced applications, allowing us to model and analyze a wide range of real-world phenomena.
In the study of differential equations, we move beyond single-variable functions and explore equations that involve derivatives or differentials. These equations describe rates of change and relationships between variables in a more intricate manner. They are used to model various physical, biological, and economic systems, such as population growth, fluid flow, and electrical circuits. Solving differential equations requires the use of techniques like separation of variables, integrating factors, and power series expansions.
Multivariable calculus, on the other hand, deals with functions of multiple variables, allowing us to analyze objects in higher dimensions. Here, we no longer work with simple curves or surfaces but instead consider functions that depend on two or more variables. This field is essential in physics, engineering, and computer science, where problems often involve multiple variables. Multivariable calculus helps us understand phenomena such as motion in three-dimensional space, optimization of functions with multiple inputs, and the behavior of vector fields.
Together, differential equations and multivariable calculus provide powerful tools for understanding and modeling complex systems. They enable us to gain insights into the behavior of dynamic systems, optimize processes, and make predictions about future outcomes. These advanced topics in calculus pave the way for further studies in mathematical analysis, physics, engineering, and other fields that require advanced mathematical techniques.
Resources for further learning in calculus
Calculus is a challenging subject that requires a solid understanding of foundational concepts and principles. Once you have completed a basic calculus course or have a grasp of the fundamentals, it can be beneficial to explore additional resources to deepen your knowledge and improve your problem-solving skills.
1. Online Courses and Tutorials
There are numerous online platforms that offer calculus courses and tutorials for individuals of all levels. Some popular options include Khan Academy, Coursera, and edX. These platforms provide comprehensive video lessons, practice problems, and interactive quizzes to help you master various calculus topics.
2. Textbooks
Textbooks are an excellent resource for learning calculus in-depth. Some highly recommended calculus textbooks include “Calculus: Early Transcendentals” by James Stewart, “Calculus” by Michael Spivak, and “Apostol: Calculus, Vol. 1” by Tom Apostol. These textbooks provide clear explanations, detailed examples, and a wide range of practice problems to enhance your understanding.
3. Online Communities and Forums
Engaging with online communities and forums can be a great way to connect with other calculus enthusiasts and experts. Websites such as MathStackExchange and Reddit have dedicated sections for calculus, where you can ask questions, participate in discussions, and gain insights from experienced individuals. These communities can provide valuable support and foster a deeper understanding of calculus concepts.
4. Practice Problems and Worksheets
Regular practice is essential for mastering calculus. There are various websites and resources that offer practice problems and worksheets for calculus. Websites like Mathway, Symbolab, and SparkNotes provide step-by-step solutions to calculus problems, allowing you to practice and reinforce your skills.
5. Tutoring and Study Groups
If you prefer a more personalized approach, consider seeking tutoring or joining a study group. Many universities and educational centers offer calculus tutoring services, either in-person or online. Collaborating with a tutor or study group can help you clarify difficult concepts, solve challenging problems, and gain valuable insights from peers and experts.
By utilizing these resources, you can continue your journey in calculus and further enhance your understanding of this fascinating branch of mathematics. Remember to stay motivated, practice regularly, and seek help when needed. With dedication and perseverance, you can become proficient in calculus and apply its principles to various real-world situations.