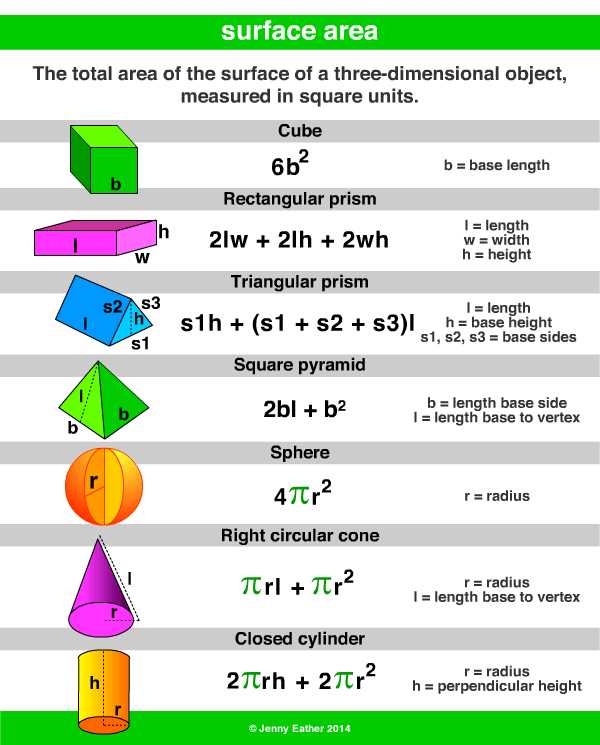
Surface area is one of the fundamental concepts in geometry and is widely used in various fields such as architecture, design, and engineering. It is the measure of the total area that the surface of an object occupies. Understanding how to calculate surface area is crucial for solving real-life problems and mathematical tasks.
Surface area task cards are a helpful tool for students to practice and reinforce their understanding of surface area calculations. These task cards typically contain different shapes, such as cubes, rectangular prisms, cylinders, and spheres, with specific dimensions provided. Students are required to determine the surface area of each shape based on the given dimensions.
An answer key is an essential component of surface area task cards as it provides the correct solutions and allows students to self-assess their work. The answer key typically includes the formulas used to calculate surface area for each shape and step-by-step solutions for each task. It serves as a valuable resource for students to check their answers and identify any mistakes they may have made during the process.
Surface Area Task Cards Answer Key
In this answer key, we will provide the solutions for the surface area task cards. We have used different formulas and techniques to calculate the surface area of various shapes and objects. Let’s dive into the answer key and find out how to solve these problems step by step.
Task Card 1: The task card asks us to find the surface area of a rectangular prism with the dimensions given as length, width, and height. To solve this problem, we will use the formula: Surface Area = 2lw + 2lh + 2wh, where l represents the length, w represents the width, and h represents the height. By plugging in the given values, we can calculate the surface area of the rectangular prism.
Task Card 2: This task card requires us to calculate the surface area of a cylinder. The formula to find the surface area of a cylinder is: Surface Area = 2πr(r + h), where r represents the radius and h represents the height of the cylinder. We will substitute the given values into the formula to find the surface area of the cylinder.
By following these techniques and using the appropriate formulas, we can find the surface area of various shapes and objects mentioned in the task cards. It is important to carefully read and understand the given dimensions and apply the correct formula to arrive at the correct answer.
- Task Card 1: Surface Area of a Rectangular Prism
- Task Card 2: Surface Area of a Cylinder
- Task Card 3: Surface Area of a Cone
- Task Card 4: Surface Area of a Sphere
Throughout this answer key, we will walk you through the process of solving these surface area problems and provide detailed explanations. Feel free to refer back to this answer key whenever you need help or clarification on how to find the surface area of different geometrical shapes.
What Are Surface Area Task Cards?
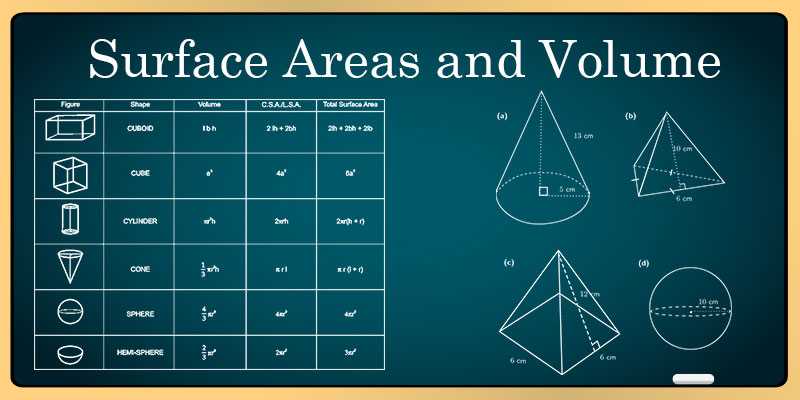
Surface area task cards are educational resources designed to help students understand and practice calculating the surface area of 3D shapes. These cards typically feature different shapes, such as cubes, rectangular prisms, cylinders, and cones, along with specific dimensions and measurements. The cards often have questions or prompts that require students to apply their knowledge of surface area formulas and calculations.
Surface area task cards are a hands-on and interactive way for students to engage with the concept of surface area. They can be used in various educational settings, including classrooms, homeschooling environments, or tutoring sessions. These task cards provide students with the opportunity to work independently or collaboratively to solve problems and deepen their understanding of surface area concepts.
Each task card usually presents a unique scenario or problem that requires students to find the surface area of a specific shape. Students must carefully analyze the given information, apply the appropriate surface area formula, and calculate the answer. These task cards can also include visual representations of the shapes, making it easier for students to visualize the concepts and develop their spatial reasoning skills.
Surface area task cards can be used as a formative assessment tool to gauge students’ understanding and progress. They can also be used for differentiation, allowing students to work at their own pace and level of difficulty. Teachers can provide support and guidance as students work through the task cards, addressing any misconceptions or difficulties that may arise.
In summary, surface area task cards are educational resources that provide students with opportunities to practice and apply their knowledge of surface area calculations. These hands-on and interactive tools help students develop their problem-solving skills, spatial reasoning, and conceptual understanding of surface area concepts.
Why Use Surface Area Task Cards?
Surface area is an important concept in geometry that is used to calculate the amount of space that is occupied by the surface of a three-dimensional object. It is an essential skill for students to master, as it is used in many real-life situations, such as measuring the amount of wrapping paper needed for a gift box or determining the amount of paint required to cover a room. To help reinforce and practice this concept, surface area task cards are an effective tool to use in the classroom.
Firstly, surface area task cards provide a hands-on and interactive approach to learning. By using task cards, students are actively engaged in the learning process and can work at their own pace. They can manipulate the cards, visualize different objects, and apply their knowledge to solve problems. This hands-on experience enhances their understanding of surface area and helps them develop their spatial reasoning skills.
Secondly, surface area task cards offer a variety of problems that cater to different learning styles and abilities. These task cards can be differentiated to meet the needs of individual students. They can be used for both independent practice and collaborative work, allowing for peer learning and discussion. Additionally, task cards can be easily modified to provide more challenging or scaffolded problems, ensuring that all students are appropriately challenged and supported.
Lastly, using task cards for surface area allows for quick and easy assessment. Teachers can observe students as they work through the problems, providing immediate feedback and identifying any misconceptions or areas of difficulty. Task cards also allow for easy tracking and documentation of student progress. They can be used as a formative assessment tool, allowing teachers to assess student understanding and adjust instruction accordingly.
In conclusion, surface area task cards are a valuable resource for teaching and learning about surface area. They provide an interactive and engaging experience, cater to different learning styles, and offer quick assessment opportunities. By incorporating task cards into the classroom, students can develop a deep understanding of surface area and apply their knowledge to real-life situations.
Tips for Using Surface Area Task Cards
Surface area task cards are a great tool for practicing and reinforcing the concept of surface area. Here are some tips to help you make the most out of using surface area task cards:
1. Familiarize Yourself with the Concepts:

Before using the task cards, it is important to familiarize yourself with the concepts of surface area. Make sure you understand how to calculate the surface area of different shapes and are comfortable with the formulas involved.
2. Start with Easy Cards:
Begin by using the easier task cards to build confidence and understanding. This will help students grasp the concept before moving on to more challenging cards. As they become more comfortable, gradually increase the difficulty level of the task cards.
3. Encourage Group Work:
Task cards can be a great way to promote collaborative learning. Encourage students to work in pairs or small groups to solve the task cards. This will allow them to discuss their thinking, share strategies, and learn from their peers.
4. Provide Manipulatives:
Using manipulatives such as cubes or nets can greatly aid in visualizing and understanding surface area. Provide students with these tools to help them visualize the different shapes and calculate the surface area accurately.
5. Use the Answer Key:
Make sure to provide students with an answer key or solution guide. This will allow them to self-check their answers and gain immediate feedback. It can also serve as a reference for students who are struggling or need extra support.
6. Discuss Real-life Applications:
Connect the concept of surface area to real-life applications to make it more meaningful and relatable for students. Discuss how surface area is used in everyday situations, such as calculating the amount of paint needed to cover a wall or determining the material required to wrap a gift box.
By following these tips, you can maximize the effectiveness of surface area task cards and help students develop a strong understanding of the concept.
Examples of Surface Area Task Card Questions
Surface area task cards are a great way for students to practice and apply their knowledge of surface area. These task cards typically present students with a picture or diagram of a three-dimensional object and ask them to find the surface area. Here are some examples of surface area task card questions:
Example 1:
A task card displays a rectangular prism with dimensions of 4 cm by 5 cm by 8 cm. The question asks students to calculate the surface area of the prism. To solve this, students need to find the area of each face and then add them together. The answer, in this case, would be 136 square centimeters.
Example 2:
Another task card shows a cylindrical container with a radius of 6 cm and a height of 10 cm. Students are asked to find the total surface area of the container, including the top and bottom parts. To solve this, students need to find the area of the base (using the formula for the area of a circle), the area of the curved surface (using the formula for the circumference of a circle and the height), and then add them together. The answer, in this case, would be 432 square centimeters.
Example 3:

A task card presents a cone with a radius of 3 cm and a slant height of 7 cm. The question asks students to determine the total surface area of the cone. To solve this, students need to find the area of the base (using the formula for the area of a circle), the area of the curved surface (using the formula for the circumference of a circle and the slant height), and then add them together. The answer, in this case, would be 111 square centimeters.
These examples demonstrate the types of questions that students may encounter on surface area task cards. By practicing with these task cards, students can strengthen their understanding of surface area and improve their problem-solving skills.
How to Use the Surface Area Task Cards Answer Key
Using the surface area task cards answer key is a straightforward process that can help students check their work and improve their understanding of the concept. By following these simple steps, students can make the most out of the answer key and enhance their learning experience.
Step 1: Solve the Task Cards
Before using the answer key, it is important for students to attempt solving the task cards on their own. This will help them practice their problem-solving skills and ensure that they are actively engaged in the learning process.
Step 2: Compare Answers
Once students have completed solving the task cards, they can compare their answers with the ones provided in the answer key. This step allows them to identify any mistakes or misconceptions they may have had while solving the problems.
Step 3: Analyze Mistakes
If students find any discrepancies between their answers and the ones in the answer key, it is important for them to analyze their mistakes. They should carefully evaluate their thought process and identify any conceptual errors or calculation mistakes they may have made.
Step 4: Seek Clarification
If students are unable to identify the mistakes or are unsure about certain concepts, it is essential for them to seek clarification from their teacher or peers. This step is crucial in deepening their understanding of surface area and ensuring that they have a clear grasp of the subject matter.
Step 5: Learn from Corrections
By analyzing their mistakes and seeking clarification, students can learn from their corrections and avoid making the same errors in the future. This process helps them improve their problem-solving skills and enhances their overall understanding of surface area.
Conclusion
The surface area task cards answer key is a valuable tool that can aid students in their learning journey. By following the steps outlined above, students can effectively use the answer key to assess their progress, identify mistakes, and enhance their understanding of surface area concepts.