Translating verbal statements into equations is a fundamental skill in algebra. It involves understanding the language of math and being able to represent real-world situations using mathematical symbols and operations. This process is essential for solving word problems and applying algebraic concepts to various scenarios.
In this article, we will provide an answer key to help you practice translating verbal statements into equations. Each question will present a verbal statement, and you will need to determine the corresponding equation. By practicing these exercises, you will enhance your algebraic skills and become more comfortable with the language of math.
To solve these problems, you will need to carefully analyze the verbal statements and identify the relevant mathematical operations. Remember to assign variables to unknown quantities and use appropriate symbols for addition, subtraction, multiplication, and division. You will also encounter a variety of keywords that indicate specific mathematical operations, such as “sum,” “difference,” “product,” and “quotient.”
Understanding Verbal Statements
Verbal statements play a crucial role in mathematics, as they often form the basis for translating real-life problems into mathematical equations. Understanding these verbal statements is essential for solving math problems effectively and accurately. In this article, we will explore some key techniques and strategies to help you understand and interpret verbal statements in mathematics.
Identifying Key Phrases:
One of the first steps in understanding a verbal statement is to identify key phrases that indicate mathematical operations or relationships. These key phrases can vary depending on the context, but some common ones include “more than,” “less than,” “sum of,” “difference between,” “product of,” and so on. By recognizing these key phrases, you can determine the mathematical operations that need to be performed.
Translating Verbal Statements into Equations:
Once you have identified the key phrases in a verbal statement, the next step is to translate them into mathematical equations. This involves assigning variables to unknown quantities and using appropriate symbols and operators to represent the given relationships. For example, if a verbal statement says “The sum of two numbers is 10,” you can represent this as an equation: x + y = 10, where x and y are the unknown numbers.
Practicing with Examples:
To improve your understanding of verbal statements in mathematics, it is important to practice with a variety of examples. Start with simple problems and gradually work your way up to more complex ones. By practicing regularly, you will become more familiar with the different types of verbal statements and the corresponding mathematical equations. Additionally, solving a wide range of examples will help you develop problem-solving skills and improve your overall mathematical proficiency.
In conclusion, understanding verbal statements is an essential skill in mathematics. By learning to identify key phrases, translating them into equations, and practicing with examples, you can enhance your ability to solve math problems accurately and efficiently. So, keep practicing and honing your skills, and soon you will become proficient in interpreting verbal statements in mathematics.
What are Verbal Statements?
Verbal statements are expressions or descriptions made using words in spoken or written form. These statements can convey information, make claims, or express opinions about a particular topic or situation. In mathematics, verbal statements often involve translating real-life problems or scenarios into mathematical equations or expressions.
When working with verbal statements in mathematics, it is important to carefully analyze the given information and identify the key elements or variables involved. This includes understanding the relationships between different quantities, identifying the operations or mathematical operations involved, and determining the desired outcome or solution.
For example, if you are given the verbal statement “Twice a number increased by five equals seventeen”, you can translate this into the equation 2x + 5 = 17, where x represents the unknown number.
In another example, if you are given the verbal statement “The length of a rectangle is three times its width”, you can translate this into the equation l = 3w, where l represents the length and w represents the width of the rectangle.
By converting verbal statements into mathematical equations, we can effectively solve problems and find solutions using mathematical techniques, such as algebraic manipulation, equation solving, or graphing.
In summary, verbal statements are descriptions or expressions made using words, and in mathematics, they can be translated into mathematical equations or expressions to solve problems or represent relationships between quantities.
Identifying Key Words
When translating verbal statements into equations, it is crucial to identify key words that represent mathematical operations or relationships. Key words can help us understand the problem and determine the appropriate mathematical equation to solve it.
Here are some key words commonly used in word problems:
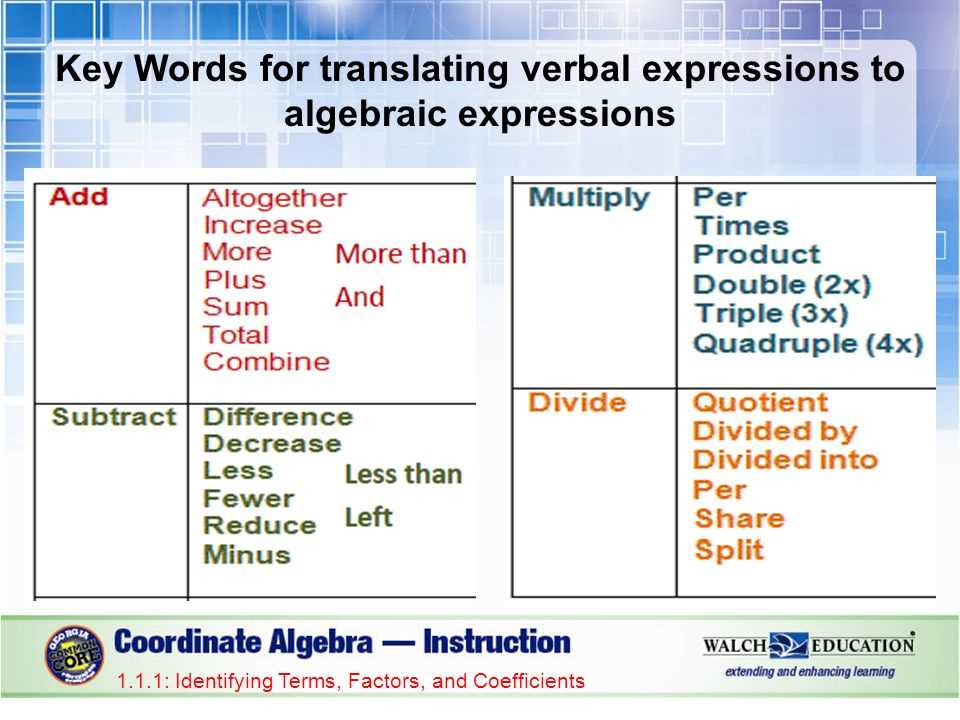
- Addition words: sum, total, increased by, plus, more than
- Subtraction words: difference, decreased by, less than, minus
- Multiplication words: product, times, twice, multiplied by
- Division words: quotient, divided by
- Equal words: is, are, was, were, gives, yields
Identifying these key words can help us represent the given information in the form of mathematical equations. For example, if we see the word “sum,” we know that we need to add the quantities mentioned. If we see the phrase “decreased by,” we know that we need to subtract. By paying attention to these key words, we can easily translate verbal statements into mathematical equations and solve the problem effectively.
Recognizing Mathematical Operations

In mathematics, it is important to be able to recognize and understand different mathematical operations. These operations include addition, subtraction, multiplication, and division. By recognizing these operations, we can translate verbal statements into equations and solve mathematical problems.
Addition: Addition is the mathematical operation of combining two or more numbers to get a total. This operation is often represented by the symbol “+”. For example, if we have two numbers, 3 and 5, the sum of these numbers would be written as 3 + 5.
Subtraction: Subtraction is the mathematical operation of taking away one number from another. This operation is often represented by the symbol “-“. For example, if we have two numbers, 8 and 4, and we subtract 4 from 8, the equation would be written as 8 – 4.
Multiplication: Multiplication is the mathematical operation of repeated addition. This operation is often represented by the symbol “x” or the asterisk “*”. For example, if we have two numbers, 2 and 3, and we want to find the product of these numbers, the equation would be written as 2 x 3 or 2 * 3.
Division: Division is the mathematical operation of splitting a number into equal parts. This operation is often represented by the symbol “÷” or the slash “/”. For example, if we have two numbers, 12 and 3, and we want to divide 12 by 3, the equation would be written as 12 ÷ 3 or 12 / 3.
By recognizing these mathematical operations and understanding how to use them, we can efficiently solve various mathematical problems and equations. It is important to practice recognizing these operations and their corresponding symbols to become more proficient in solving mathematical equations.
Translating Verbal Statements into Equations
When solving mathematical problems, it is often necessary to translate verbal statements into equations. This process involves understanding the problem, identifying the key information, and representing it mathematically.
In order to translate verbal statements into equations, it is important to pay attention to the keywords and phrases that indicate mathematical operations. For example, words like “sum,” “difference,” “product,” and “quotient” indicate addition, subtraction, multiplication, and division, respectively.
Let’s take an example to illustrate this process. Suppose we have a problem that states: “Three times a number increased by five is equal to eight.” To translate this into an equation, we can first identify the key information: “three times a number” and “increased by five is equal to eight.” We can represent the unknown number with a variable, let’s say x.
Using the information given, we can write the equation: 3x + 5 = 8. Here, the phrase “three times a number” is translated to 3x, and “increased by five” is represented by +5. The equation states that the sum of 3x and 5 is equal to 8.
Translating verbal statements into equations allows us to solve mathematical problems using algebraic methods. By properly identifying key information and representing it mathematically, we can effectively solve complex problems and find solutions to real-world situations.
Using Variables

Variables play an essential role in translating verbal statements into equations. They allow us to represent unknown quantities and solve problems algebraically. By assigning a letter or symbol to represent an unknown value, we can create equations that express relationships between different quantities.
For example, let’s say we have an unknown number that we want to represent with the variable “x”. We can then create an equation like “2x + 5 = 15”, where “2x” represents twice the value of “x” and “5” is a constant. By solving this equation, we can find the value of “x” and determine the unknown number.
Variables are also useful when dealing with mathematical word problems. For instance, if we have a word problem that states, “John is three times older than his sister Sarah, and the sum of their ages is 32”, we can assign variables to represent their ages. Let’s say “x” represents Sarah’s age. Then we can translate the problem into the equation “3x + x = 32”, where “3x” represents three times Sarah’s age and “x” represents her age. By solving this equation, we can find the ages of both John and Sarah.
Using variables allows us to generalize mathematical statements and solve a wide range of problems. They provide a way to represent unknown quantities and express relationships between them in a concise and symbolic manner. So whether we’re solving equations or tackling word problems, variables are an essential tool for translating verbal statements into equations.
Solving Verbal Equations
Solving verbal equations involves translating verbal statements into mathematical equations and then solving for the unknown variable. Verbal equations are word problems that require the use of algebra to find the answer. They often involve setting up an equation based on the information given in the problem and then performing the necessary operations to find the solution.
When solving verbal equations, it is important to carefully read and understand the problem. Look for keywords and phrases that indicate mathematical operations such as addition, subtraction, multiplication, or division. These keywords will help in translating the problem into an equation.
For example, if the problem states “Twice a number increased by 5 equals 15,” we can translate it into the equation 2x + 5 = 15, where x represents the unknown number. By solving this equation, we can find the value of x, which in this case is 5.
It is also necessary to carefully consider the units mentioned in the problem. For instance, if the problem involves distance or time, make sure to convert units if needed before setting up the equation. This will ensure that the equation accurately represents the problem and leads to the correct solution.
Solving verbal equations is an essential skill in algebra and can be applied to a variety of real-life situations. It requires logical reasoning, problem-solving abilities, and a solid understanding of algebraic concepts. By practicing translating verbal statements into equations and solving them, you will improve your overall mathematical skills and problem-solving abilities.
Applying Algebraic Techniques
In this article, we have discussed the process of translating verbal statements into equations. By following a step-by-step approach and consistently identifying variables and translating the given information into mathematical expressions, we can effectively solve problems using algebraic techniques.
Throughout the examples provided, we have seen how to translate various types of verbal statements, such as sentences describing the relationship between quantities, into algebraic equations. We have also learned how to solve these equations to find the values of the variables that satisfy the given conditions.
By applying algebraic techniques, we can solve a wide range of problems in various fields, including mathematics, physics, economics, and engineering. These techniques provide a systematic and logical approach to problem-solving, allowing us to solve complex problems with ease.
- We start by identifying the variables and assigning them appropriate names.
- We then translate the given information into algebraic expressions using appropriate symbols and operators.
- We set up equations by equating the expressions on either side of the equal sign.
- We solve these equations to find the values of the variables.
By consistently following these steps, we can effectively apply algebraic techniques to solve a variety of problems. It is important to practice and familiarize oneself with the process of translating verbal statements into equations, as it is a fundamental skill in mathematics and problem-solving.
Overall, understanding how to translate verbal statements into equations and applying algebraic techniques is crucial for success in algebra and other mathematical disciplines. By mastering these skills, we can confidently tackle complex problems and find solutions using the power of algebra.