In mathematics, linear relationships are a fundamental concept. They form the building blocks for more complex concepts like algebra and calculus. Unit 3 focuses on understanding and working with linear relationships, and this article provides an answer key to the unit’s exercises and problems.
In this unit, you will learn how to graph and interpret linear functions, solve equations, and analyze the slope and y-intercept of a line. You will also learn about the different forms of a linear equation, such as slope-intercept form and point-slope form. By the end of the unit, you will be able to solve real-world problems using linear relationships.
The answer key provided in this article will serve as a helpful guide for checking your work and understanding the solutions. It includes step-by-step explanations and examples for each exercise and problem in Unit 3. Whether you are a student studying linear relationships or a teacher looking for an answer key, this article will provide you with the resources you need to succeed.
Understanding Linear Relationships: Unit 3 Answer Key
Unit 3 of the linear relationships curriculum focuses on understanding the concept of linear relationships and how to analyze and solve problems involving linear equations and inequalities. This answer key provides a comprehensive list of answers to the exercises and questions in the unit, allowing students to check their work and fully understand the material.
The answer key includes solutions to various types of problems, such as finding the slope and y-intercept of a line, graphing linear equations and inequalities, and solving word problems using linear equations. It also provides step-by-step explanations and diagrams to help students visualize the concepts being taught.
By using the answer key, students can independently review and assess their progress in the unit. They can compare their answers to the correct solutions provided and identify any mistakes or areas of weakness. This allows them to focus on specific topics that require further practice or clarification.
The answer key serves as a valuable tool for both students and educators. It promotes self-directed learning and provides immediate feedback, helping students take ownership of their learning process. Educators can also use the answer key as a resource for grading assignments and assessing student comprehension.
Overall, the “Understanding Linear Relationships: Unit 3 Answer Key” is an essential companion for students studying linear relationships. It facilitates comprehension, self-assessment, and helps develop problem-solving skills necessary for success in mathematics.
Exploring Linear Equations and Functions
Linear equations and functions are fundamental concepts in mathematics that provide a powerful tool to model and analyze real-world situations. They are used to describe relationships between variables that have a constant rate of change. By exploring linear equations and functions, we can gain insights into various aspects of our world, such as economics, physics, engineering, and more.
One key idea in linear equations is the concept of slope, which represents the rate of change between two variables. The slope tells us how one variable changes as the other variable changes. It can be positive, negative, or zero, indicating a steep increase, a steep decrease, or no change at all. The slope-intercept form of a linear equation, y = mx + b, shows the relationship between the dependent variable y and the independent variable x, where m represents the slope and b represents the y-intercept.
When exploring linear equations and functions, it is helpful to graph them on a coordinate plane. By plotting points and connecting them with a straight line, we can visualize the relationship between the variables. The graph of a linear function is always a straight line, and its slope determines the steepness of the line. We can also use the graph to find the x-intercept and the y-intercept, which are the points where the line crosses the x-axis and y-axis, respectively.
In addition to graphing linear equations and functions, we can also solve them algebraically. This involves manipulating the equation to isolate the variable of interest. By solving for the variable, we can find specific values or determine the conditions for which the equation is true. We can also use algebraic methods to analyze the solutions and interpret them in the context of the problem.
Overall, exploring linear equations and functions allows us to uncover patterns, make predictions, and solve real-world problems. By understanding the relationship between variables and how they change, we can gain valuable insights and make informed decisions in various fields. Whether it’s analyzing economic data, designing engineering systems, or understanding the motion of objects, linear equations and functions are indispensable tools in our mathematical toolkit.
Solving Linear Equations
Linear equations are mathematical equations that involve only variables to the power of 1. They can be solved by isolating the variable and finding its value. Solving linear equations is an essential skill in algebra and is used in various real-life applications.
One way to solve a linear equation is by using the properties of equality. The goal is to isolate the variable on one side of the equation. This is done by performing the same operation on both sides of the equation, which maintains the equality. For example, if we have the equation 2x + 5 = 13, we can subtract 5 from both sides to get 2x = 8. Then, we divide both sides by 2 to solve for x, which gives us x = 4.
Another method to solve linear equations is by using the concept of slope-intercept form. This form is written as y = mx + b, where m represents the slope and b represents the y-intercept. By rearranging the equation to isolate y, we can solve for x. For example, if we have the equation 3x – 2y = 6, we can rewrite it as -2y = -3x + 6. Dividing both sides by -2 gives us y = (3/2)x – 3. In this form, we can easily determine the slope and y-intercept, which helps us graph the equation.
Solving linear equations is a fundamental skill that allows us to find the unknown values in various mathematical and real-life problems. It is important to understand the different methods and approaches to solve these equations, as they can be applied to a wide range of situations, from calculating distances to determining rates of change.
Graphing Linear Equations

Graphing linear equations is a fundamental concept in algebra that involves plotting points on a graph to create a visual representation of a relationship between two variables. These variables are typically represented on the x and y axes, with the x axis representing the independent variable and the y axis representing the dependent variable.
To graph a linear equation, we need to first determine its slope-intercept form, which is written as y = mx + b, where m represents the slope of the line and b represents the y-intercept. The slope of a line determines its steepness, while the y-intercept represents the point where the line crosses the y axis.
Once we have the slope-intercept form of the linear equation, we can plot points on the graph by choosing values for the x variable and using the equation to find the corresponding y values. By connecting these points with a straight line, we can visualize the relationship between the variables and determine if it is positive or negative.
A positive slope means that as the x values increase, the y values also increase, creating an upward-sloping line. On the other hand, a negative slope means that as the x values increase, the y values decrease, resulting in a downward-sloping line. A slope of zero indicates a horizontal line, while an undefined slope represents a vertical line.
By graphing linear equations, we can analyze and understand the relationship between variables and make predictions based on the patterns observed. This visual representation allows us to easily interpret and communicate mathematical concepts, making graphing an essential skill in algebra and beyond.
Interpreting Graphs of Linear Equations

When studying linear equations, it is essential to understand how to interpret graphs representing these equations. Graphs can provide valuable information about the relationship between variables and help us make predictions or analyze patterns.
A linear equation is represented by a straight line on a graph, and its slope and y-intercept can be determined by analyzing the graph. The slope of a line indicates how steep it is, while the y-intercept represents the point where the line crosses the y-axis. By examining these characteristics, we can gain insight into the behavior of the equation.
Example: Let’s consider the equation y = 2x + 3. The slope of the line is 2, which means that for every unit increase in x, the corresponding y-value increases by 2. The y-intercept is 3, indicating that the line crosses the y-axis at the point (0,3).
Graphs can also help us identify the relationship between two variables. If the line slopes upward from left to right, it indicates a positive relationship: as one variable increases, so does the other. Conversely, if the line slopes downward, there is a negative relationship: as one variable increases, the other decreases.
Furthermore, the steepness of the line can give us information about the strength of the relationship. A steeper line suggests a stronger relationship, while a flatter line indicates a weaker one. By analyzing these characteristics, we can draw conclusions about the variables and make predictions about their behavior.
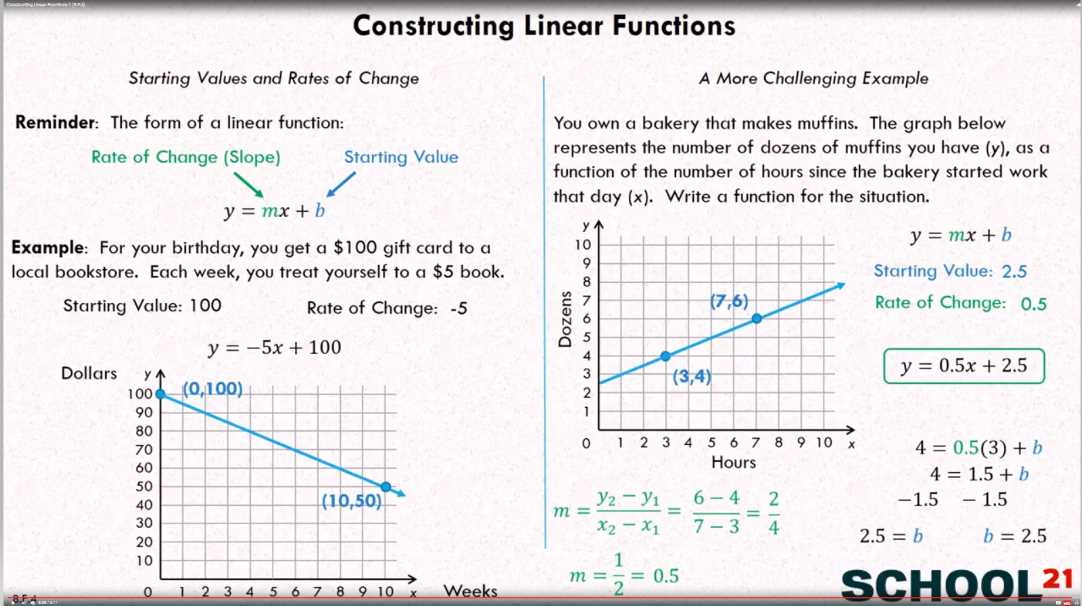
Slope and Rate of Change
The concept of slope and rate of change is fundamental in linear relationships. Slope represents how steep a line is, and rate of change measures how one variable changes in relation to another. In a linear equation of the form y = mx + b, where m is the slope and b is the y-intercept, the slope is a ratio that tells us how much y changes for every unit change in x. It can be positive, negative, or zero.
To calculate the slope, we can pick any two points on the line and use the formula: slope = (y2 – y1) / (x2 – x1). The numerator represents the change in y, and the denominator represents the change in x. For example, if a line has a slope of 2/3, it means that for every 1 unit increase in x, y increases by 2/3 units.
The rate of change is closely related to slope. It measures how one quantity changes with respect to another. For example, in a real-life scenario, if we are looking at the relationship between distance and time, the rate of change would represent the speed at which an object is moving. If the rate of change is constant, it means the relationship is linear. If the rate of change varies, the relationship may be nonlinear or involve multiple factors.
Understanding slope and rate of change is crucial in various fields, such as physics, economics, and mathematics. It allows us to analyze and interpret data, make predictions, and model real-life situations. By calculating the slope and rate of change, we can gain valuable insights into the patterns and trends in linear relationships.
Analyzing Linear Relationships

In this unit, we have explored the concept of linear relationships. We have learned how to represent linear relationships using equations, graphs, and tables. We have also learned how to find the slope and y-intercept of a line and how to write the equation of a line in slope-intercept form.
By analyzing linear relationships, we can determine if two variables are related and how they are related. We can use the slope to determine the direction and steepness of the line, and the y-intercept to determine the starting point of the line. We can also use the equation of a line to make predictions and solve problems.
Throughout this unit, we have practiced analyzing linear relationships through various examples and exercises. We have learned how to calculate slopes and y-intercepts, how to interpret graphs and tables, and how to write and solve equations. By mastering these skills, we have gained a deeper understanding of linear relationships and how they can be applied in real-world situations.
Overall, analyzing linear relationships is a valuable skill that can be used in many areas of math and science. It allows us to better understand and interpret data, make predictions, and solve problems. It is an important foundation for further studies in algebra and calculus.