Geometry is filled with intricate relationships between shapes and figures. In particular, understanding proportionality and the relationships between different parts of a figure is crucial for solving problems efficiently. This section provides an essential overview of these core principles, helping you navigate complex calculations and reasoning challenges.
By focusing on essential techniques and methods, you will be equipped to tackle a wide range of questions with confidence. This material not only enhances your ability to solve problems but also strengthens your understanding of fundamental geometric relationships. Mastering these concepts will lay the groundwork for excelling in upcoming evaluations and exams.
Prepare yourself for the challenges ahead by reviewing these key points carefully. By practicing regularly and engaging with the material actively, you can achieve mastery and enhance your performance significantly.
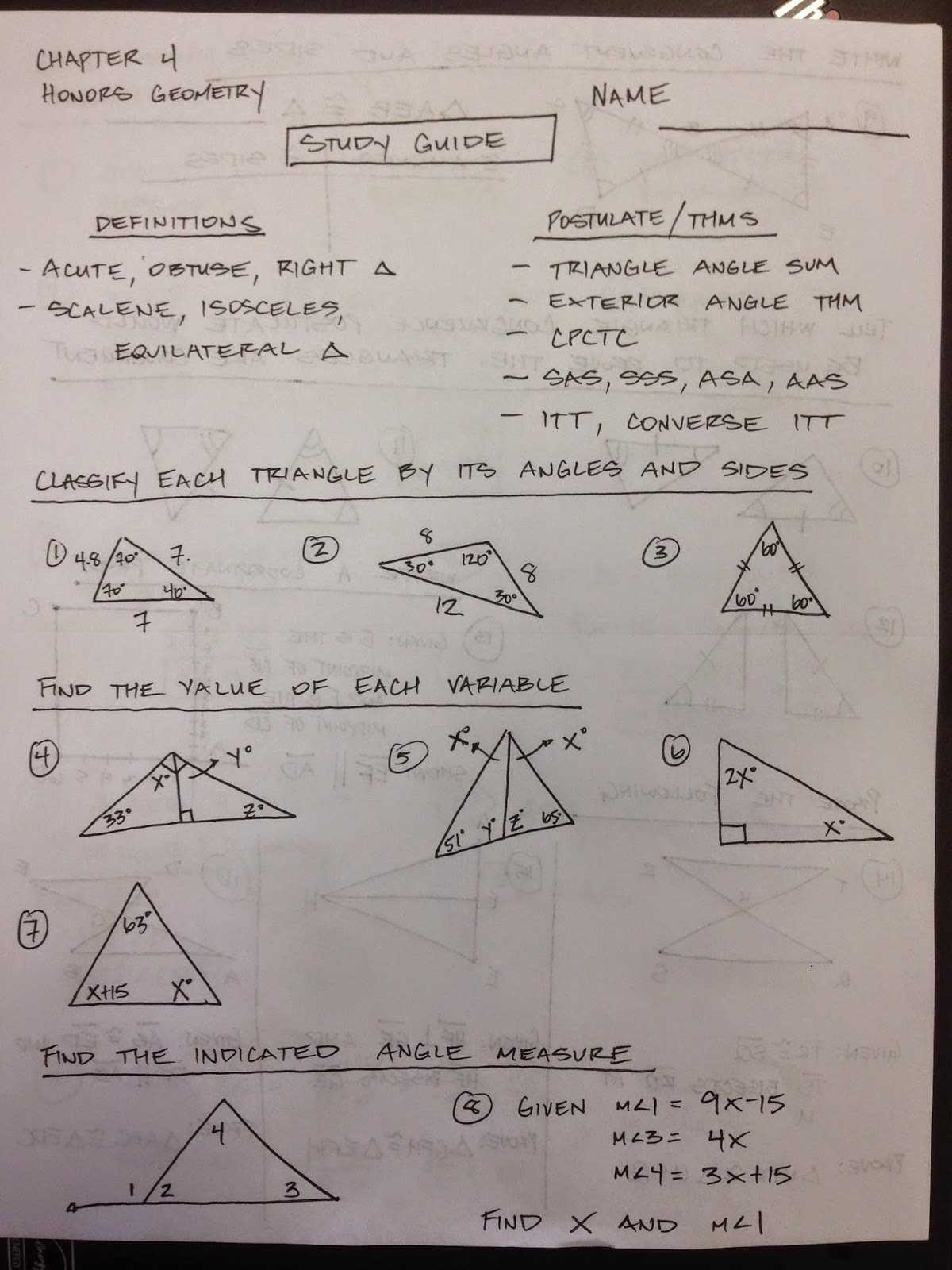
Understanding Geometric Proportions Basics
In geometry, recognizing the relationships between various shapes is key to solving a wide array of problems. When working with proportionality, it’s important to grasp how different figures share common properties that allow for comparisons and calculations. This section introduces the foundational concepts that help in identifying and solving problems involving proportional shapes.
Two shapes are said to have proportional sides and corresponding angles, making it possible to solve for unknown parts using a variety of methods. The main idea is that if two objects share similar proportions, their corresponding elements maintain a consistent ratio.
- Proportional sides follow specific ratios across corresponding parts.
- Matching angles are equal, regardless of size differences.
- Mathematical techniques allow for accurate calculations using these relationships.
Understanding these principles is vital for making accurate predictions and calculations in geometry. The next step is to examine how these proportions translate into practical applications and problem-solving techniques.
Key Concepts for Effective Geometry Problem Solving
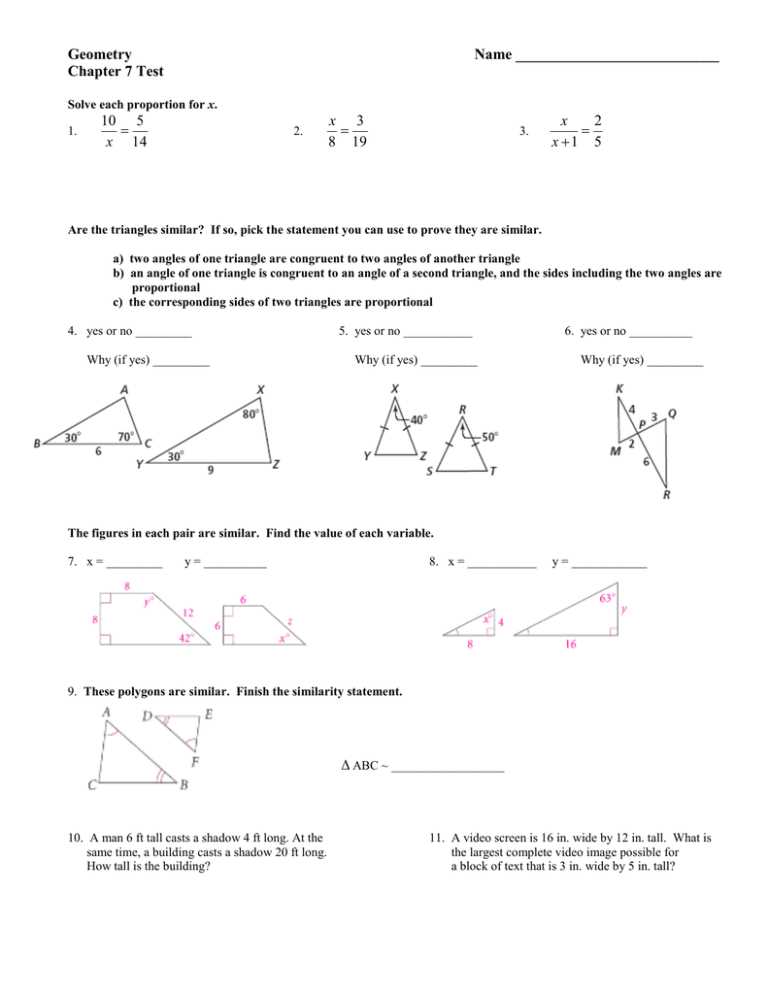
In this section, we focus on the fundamental principles that are crucial for approaching and solving geometry problems involving proportional figures. Mastery of these core concepts will not only help you understand the material more deeply but also improve your ability to apply strategies for solving complex problems effectively.
Proportions and Ratios in Geometry

Understanding proportions is essential for comparing different parts of shapes. The ratio of corresponding sides is key to determining the relationships between figures. Once you establish these ratios, solving for unknown values becomes straightforward.
Corresponding Angles and Their Significance
Another important concept is recognizing corresponding angles. These angles are equal when two figures share proportional sides, making it easier to set up equations and determine missing measurements.
By mastering these fundamental principles, you will be better equipped to solve geometry problems that involve comparisons between shapes and their parts. Familiarity with these concepts ensures more efficient problem-solving and better test performance.
How to Identify Proportional Geometric Figures
Recognizing when two shapes share proportional relationships is a key skill in geometry. By identifying certain characteristics, you can determine if two figures have matching proportions and similar properties, making them easier to work with in problem-solving scenarios.
Check for Equal Corresponding Angles
One of the first steps is to verify that the corresponding angles between two figures are identical. If the angles match, it’s a strong indication that the figures may share proportional sides, which is a hallmark of having similar properties.
Verify the Ratio of Corresponding Sides
The next step is to compare the sides of the figures. If the ratios of corresponding sides are consistent, this confirms that the figures are proportionally related. This relationship allows for further calculations to solve for unknowns in both figures.
By applying these checks, you can confidently identify figures that share proportional relationships and proceed with the appropriate mathematical methods to solve for missing elements.
Essential Formulas for Geometric Proportions
In geometry, having the right formulas at your disposal is essential for solving problems involving proportional relationships. These formulas allow you to calculate missing lengths, angles, and other measurements by understanding how different parts of a shape relate to each other.
Below is a table that highlights some of the most important formulas used to solve proportional problems involving geometric figures:
| Formula | Description |
|---|---|
| Ratio of Corresponding Sides | The ratio of two corresponding sides in proportional figures is constant. |
| Angle-Angle (AA) Postulate | If two corresponding angles are equal, the figures are proportional. |
| Side-Angle-Side (SAS) Theorem | If one pair of sides are proportional and the included angle is the same, the figures are proportional. |
| Side-Side-Side (SSS) Theorem | If all corresponding sides of two figures are proportional, the figures are proportional. |
By applying these formulas, you can easily determine the proportionality of figures and solve for unknown values, ensuring you have a strong foundation for tackling more complex problems.
Common Mistakes in Proportional Figures

When solving problems involving proportional shapes, it’s easy to make mistakes that can lead to incorrect answers. Understanding and avoiding these common errors is key to mastering the concepts and performing well in geometry.
One frequent mistake is failing to properly identify corresponding sides or angles. Mislabeling parts of the figures can result in using the wrong ratios, which leads to incorrect calculations. Another common issue arises when students incorrectly assume two shapes are proportional without checking all necessary properties, such as equal angles and consistent side ratios.
Additionally, some students mistakenly apply formulas or theorems that are not relevant to the specific problem. It’s crucial to match the correct method to the problem type, ensuring accurate results.
By staying aware of these errors and carefully reviewing each step, you can avoid pitfalls and improve your ability to solve geometric problems effectively.
Tips for Effective Test Preparation
Preparing for an assessment requires a clear strategy and focused effort. The key to success is consistent practice, understanding the underlying principles, and reviewing material in a structured way. By following effective preparation techniques, you can boost your confidence and perform well on evaluations.
Practice with Sample Problems

One of the most effective ways to prepare is by solving practice problems. This helps you get familiar with the types of questions you’ll encounter and gives you the opportunity to apply what you’ve learned. Regular practice also helps reinforce concepts and improves your problem-solving skills.
Review Mistakes and Clarify Concepts
After working through practice problems, take the time to review any mistakes. Understanding where you went wrong and clarifying concepts will deepen your comprehension and prevent similar errors on the actual evaluation. Don’t hesitate to seek clarification for any topics you’re unsure about.
By using these strategies, you can optimize your preparation and approach the assessment with a sense of readiness and clarity.