
In Unit 7 of our mathematics curriculum, we delve into the world of polynomials and factoring. This unit focuses on understanding the different types of polynomials, their properties, and how to factor them. Homework 10 is an important part of this unit, as it provides students with the opportunity to apply their knowledge and skills.
The answer key for Homework 10 is a valuable resource for both students and teachers. It allows students to check their work, identify any mistakes, and learn from them. For teachers, the answer key serves as a guide for assessing student understanding and providing feedback. Moreover, the answer key helps teachers identify common misconceptions and address them in future lessons.
By using the answer key, students can build their confidence and develop a deeper understanding of polynomials and factoring. They can also gain insight into different problem-solving strategies and learn from the approaches used by their peers. Overall, the answer key for Homework 10 is a useful tool that supports student learning and facilitates effective teaching.
Unit 7 Polynomials and Factoring Homework 10 Answer Key
In Unit 7 of our math course, we have been studying polynomials and factoring. This homework assignment, titled “Homework 10,” provided an opportunity for students to apply their knowledge and skills in these areas. The answer key for this homework assignment will help students understand and review their work, as well as identify any areas where they may need additional practice or support.
The answer key for Homework 10 includes step-by-step solutions for each problem. It provides a clear breakdown of the problem-solving process, allowing students to follow along and check their own work. In addition, it offers explanations and reasoning behind each step, helping students grasp the underlying concepts and strategies involved in solving polynomial and factoring problems.
Some of the key topics covered in Homework 10 include factoring quadratic expressions, factoring the difference of squares, factoring special products, and solving polynomial equations by factoring. The answer key not only provides the correct answers but also offers alternative approaches and strategies for solving each problem. This allows students to explore different methods and choose the one that they find most effective.
By using the Unit 7 Polynomials and Factoring Homework 10 Answer Key, students can review and reinforce their understanding of the concepts and skills covered in the unit. They can check their work, identify any errors or misunderstandings, and seek additional help or practice as needed. With consistent practice and review, students can build confidence and proficiency in working with polynomials and factoring, setting a solid foundation for future math studies.
Overview

In Unit 7 of the course, we will be focusing on polynomials and factoring. This unit is an important building block in algebra and lays the foundation for many advanced concepts in mathematics. Understanding polynomials and factoring is crucial as it allows us to solve equations, find zeros, and simplify expressions.
During this unit, we will cover various topics related to polynomials and factoring. We will start by reviewing the basic terms and concepts, such as degree, coefficient, and types of polynomials. We will then move on to exploring operations with polynomials, including addition, subtraction, multiplication, and division.
Factoring is a major topic in this unit. We will learn different methods for factoring polynomials, such as factoring by grouping, factoring trinomials, and factoring by using special patterns. Factoring is a key skill in algebra as it allows us to solve polynomial equations, find the zeros of a polynomial, and simplify complex expressions.
Throughout this unit, we will practice solving various problems and exercises to solidify our understanding of polynomials and factoring. We will also learn how to apply these concepts to real-life situations and problem-solving scenarios. By the end of this unit, we should be confident in our ability to work with polynomials, factor them, and use them to solve equations and simplify expressions.
Understanding Polynomials
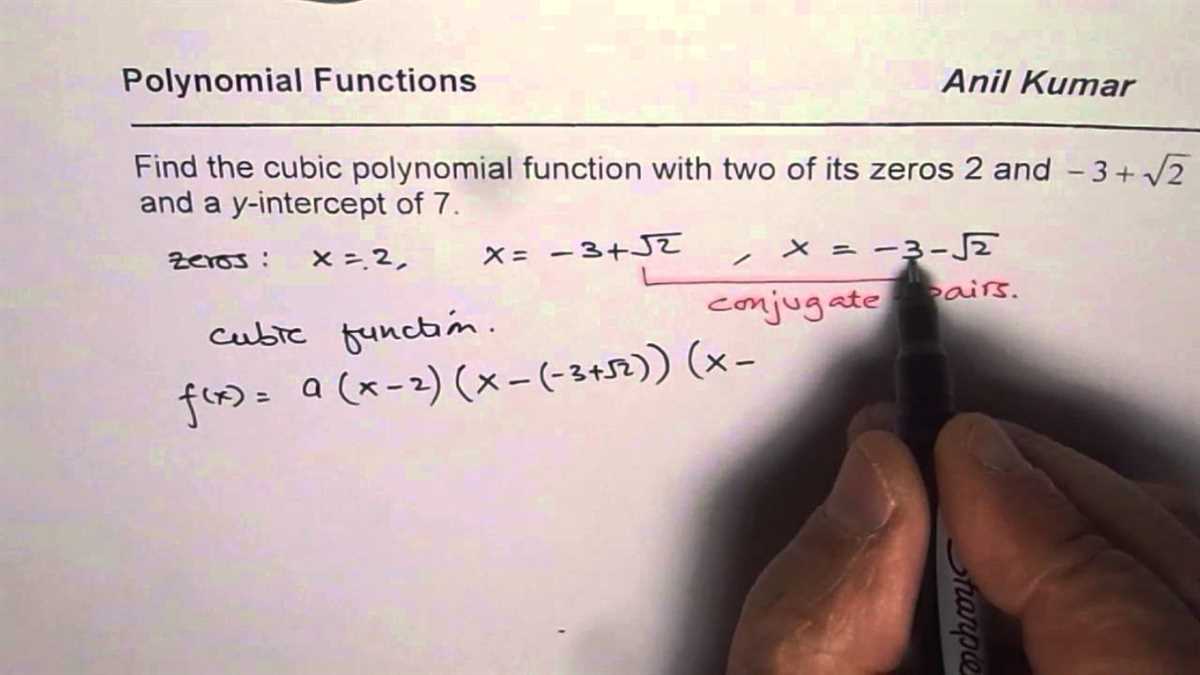
Polynomials are mathematical expressions that consist of variables and coefficients, as well as addition, subtraction, and multiplication operations. They are an essential concept in algebra and play a crucial role in many areas of mathematics and physics.
A polynomial can have one or more terms, with each term containing a variable raised to a non-negative integer exponent, multiplied by a coefficient. For example, the polynomial 3x^2 + 2xy – 5 consists of three terms: 3x^2, 2xy, and -5. The variables x and y are raised to the exponents 2 and 1, respectively.
Polynomial degree
The degree of a polynomial is determined by the highest exponent of the variable in the polynomial. For example, in the polynomial 3x^2 + 2xy – 5, the highest exponent is 2, so the polynomial is of degree 2. The degree of a polynomial can help determine its behavior and properties.
Operations with polynomials
Polynomials can be added, subtracted, and multiplied, just like any other algebraic expression. Adding and subtracting polynomials involves combining like terms, which means adding or subtracting the coefficients of the same variable raised to the same exponent.
Multiplying polynomials follows the distributive property, where each term of one polynomial is multiplied by each term of the other polynomial. The resulting polynomial is obtained by combining like terms and simplifying the expression.
To summarize, polynomials are mathematical expressions that consist of variables, coefficients, and operations. Understanding their degree and how to perform operations with them is essential in algebra and beyond.
Advantages of Factoring
Factoring is an essential tool in algebra that offers several advantages for solving polynomial equations and simplifying complex expressions. By breaking down a polynomial into its factors, mathematicians can gain valuable insights into the equation’s properties and make it easier to manipulate and solve. Here are some key advantages of factoring:
- Simplification: Factoring enables the reduction of complex expressions into simpler forms. By breaking down a polynomial into its factors, long and convoluted equations can be transformed into more manageable and understandable forms. This simplification process allows for easier analysis and manipulation of the equation.
- Solving Equations: Factoring is a powerful technique for solving polynomial equations. By factoring the equation into its constituent parts, mathematicians can identify the roots or solutions of the equation more easily. This approach is particularly useful for quadratic equations and higher-order polynomials, where factoring allows for a more straightforward solution.
- Identifying Patterns: Factoring can help identify patterns and relationships within an equation. By examining the factors of a polynomial, mathematicians can uncover crucial information about its behavior, such as the presence of repeated roots or the existence of symmetry. These insights can provide a deeper understanding of the equation’s properties and aid in further mathematical exploration.
- Efficient Computation: Factoring allows for more efficient computation, especially when dealing with large and complex expressions. Instead of manually expanding and manipulating polynomials, factoring enables mathematicians to work with the smaller factors, reducing the number of calculations required and saving time and effort.
In conclusion, factoring is a valuable technique in algebra that offers numerous advantages for simplifying equations, solving polynomial equations, identifying patterns, and improving computational efficiency. By breaking down a polynomial into its factors, mathematicians can gain valuable insights into the equation’s properties and make it easier to manipulate and analyze. Factoring is an essential tool in the mathematician’s arsenal, enabling them to solve complex problems and uncover hidden relationships within equations.
Common Factoring Techniques
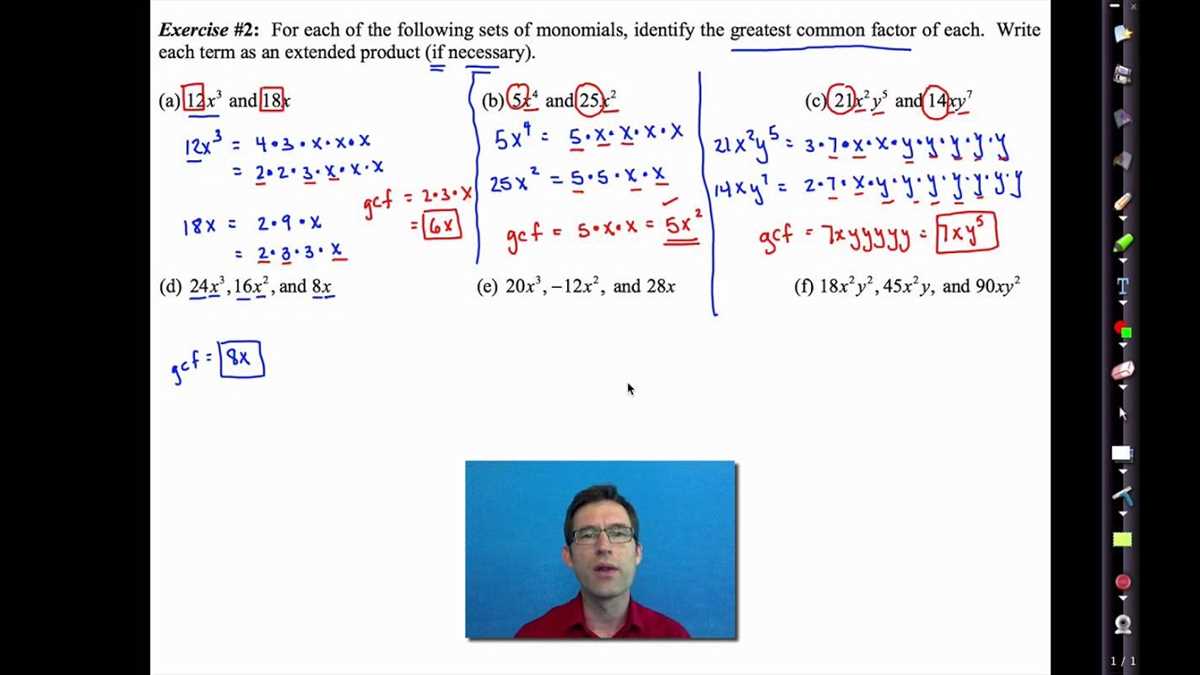
Factoring is an important skill that is used in algebra to simplify and solve equations. Common factoring techniques include factoring out the greatest common factor (GCF), factoring by grouping, and factoring trinomials. These techniques allow us to break down complex polynomials into simpler forms, making them easier to work with.
Factoring out the greatest common factor: When factoring out the GCF, we look for the largest factor that is common to all the terms in the polynomial. We then divide each term by this factor and rewrite the polynomial as a product of the GCF and the remaining terms. This simplifies the polynomial and allows us to further factorize it if necessary.
Factoring by grouping: Sometimes, a polynomial can be factored by grouping the terms into pairs and finding common factors within each pair. We then factor out the common factors, which leaves us with two smaller expressions that can be further factored if possible.
Factoring trinomials: Trinomials are polynomials with three terms. To factor trinomials, we look for pairs of numbers that multiply to give the constant term, and add up to give the coefficient of the middle term. By finding these “magic” numbers, we can rewrite the trinomial as a product of two binomials.
These common factoring techniques are fundamental in algebra and provide a foundation for solving equations and simplifying expressions. Familiarity with these techniques can greatly facilitate problem-solving and help develop a deeper understanding of polynomial operations.
Evaluating Polynomials
Polynomials are algebraic expressions that consist of variables, coefficients, and exponents. They are widely used in various mathematical and scientific fields. Evaluating polynomials involves substituting specific values for the variables in the expression and simplifying the resulting expression.
When evaluating a polynomial, it is important to follow the order of operations, starting with any operations inside parentheses or brackets, then evaluating exponents, and lastly performing addition and subtraction. The goal is to simplify the expression as much as possible.
To evaluate a polynomial, start by substituting the given values for each variable in the expression. For example, if the polynomial is 3x^2 + 2x – 5 and the value of x is 4, substitute 4 for x: 3(4)^2 + 2(4) – 5. Then, perform the operations to obtain the final result.
Polynomials can also be evaluated for multiple values of the variable, creating a table of values. This can be helpful in identifying patterns and understanding the behavior of the polynomial for different inputs. The table of values can be created by substituting different values for the variable and evaluating the expression for each value.
Evaluating polynomials is an essential skill in algebra and is used in a variety of applications, such as solving equations, graphing functions, and analyzing mathematical models. It allows us to understand the behavior of polynomial functions and make predictions based on their values.
Applications of Polynomials and Factoring

In this article, we have explored the concepts of polynomials and factoring, and how they can be applied in various real-life situations. Polynomials are mathematical expressions that involve variables, coefficients, and exponents, and they are used extensively in fields such as physics, engineering, economics, and computer science.
We have seen how to add, subtract, multiply, and divide polynomials, as well as how to simplify and factor them. These skills are essential in solving equations, graphing functions, and analyzing data. Polynomials and factoring are also used in modeling real-world phenomena, such as population growth, projectile motion, and financial investments.
Some specific applications of polynomials and factoring include:
- Calculating areas and volumes: Polynomials are used to represent and calculate areas of geometric shapes, such as rectangles, triangles, and circles. Similarly, polynomials can be used to find the volume of three-dimensional objects, such as cylinders and spheres.
- Optimization problems: Factoring polynomials can help solve optimization problems, where the goal is to find the maximum or minimum value of a function. By factoring the function, we can identify the critical points and make informed decisions.
- Engineering design: Polynomials are used in engineering design to model and analyze various physical phenomena, such as structural stability, fluid flow, and electrical circuits. Factoring allows engineers to identify key factors that affect the performance of a design and make necessary improvements.
In conclusion, polynomials and factoring have wide-ranging applications in numerous fields. They are powerful tools for problem-solving, modeling, and analysis, and understanding their concepts and techniques is crucial for success in mathematics and beyond.